
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Director

Joined: 16 May 2014
Posts: 592
Given Kudos: 0
GRE 1: Q165 V161
Facts about Ordinary Triangles on the GRE
[#permalink]
 15 Sep 2014, 14:15
15 Sep 2014, 14:15
2
Expert Reply
Question Stats:
0% (00:00) correct 0% (00:00) wrong
0% (00:00) wrong  based on 0 sessions
based on 0 sessions
Hide Show timer Statistics
Often, when we talk about triangles in the context of the GRE, we focus on right triangles (a relatively elite category) or the special right triangles (viz. 30-60-90 and 45-45-90 triangles, a hyper-elite category). Elsewhere I have written of that most glorious theorem, the Pythagorean Theorem, which applies to all right triangles.
But just as there are many more people who don’t live in the Hamptons than do, just as there are many more former Major League Baseball players who aren’t in the Baseball Hall of Fame then those who are, so there are many more non-right triangles than right triangles. This is a post about them, about the 99% of triangles. What’s true, not just for elite triangle, but for all triangles?
Well, first of all, the sum of the three angles of any triangle is 180º. Everyone knows that already. A couple cool consequences:
(1) if all three angles are equal (an equiangular, equilateral triangle), then all three angles must be 180º/3 = 60º;
(2) in a right triangle, the sum of the two acute angles must be 90º.
Both are good fact to have at your fingertips on the GMAT.But, there we go, with the elite folks again. Back to everyone else.
That has a scary sounding name, doesn’t it? It sounds really sophisticated. In fact, it’s an idea so simple that a small child could understand the basics. Formally, it says: the sum of any two sides of a triangle must be greater than the third. In picture form:

11.JPG [ 17.77 KiB | Viewed 7451 times ]
The Triangle Inequality produces these three inequalities for any triangle. Now, think about why this has to be true. The shortest distance between point A and point C has to be the straight line: this is the piece where we’d expect a little kid to understand. If we take a “crooked” path from A to C (via point B), that has to be longer than taking the straight line distance, represented by AC. That’s the simple idea at the heart of the Triangle Inequality.
Just as a political discussion of “the 99%” inevitably involves issues of socioeconomic inequality, so too, even when we leave the elite triangles and wander through the great unwashed masses of ordinary triangles, we run into geometric inequalities. This is another.
The Side Angle Inequalities. First, the technical way to say it: the measures of the three angles of a triangle are unequal in the same order as the lengths of theiropposite sides. That’s difficult to understand. Next, a picture:
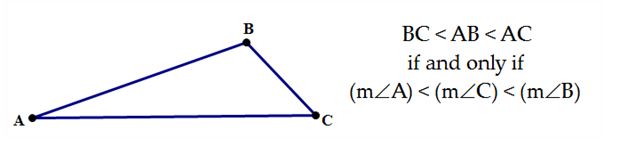
12.JPG [ 17.14 KiB | Viewed 7422 times ]
Now, the really down-to-earth, straightforward way to say the idea is: the biggest side is always opposite the biggest angle, and the smallest side is always opposite the smallest angle. (In fact, they only reason I mentioned those other complicated ways to say it was to give you the challenge of figuring out why they just say the same thing as the simple bold statement.)
Those two mathematical patterns, the Triangle Inequality and the Side-Angle Inequalities, are true for all triangles — both the elite and not-elite triangles. On GRE Math, these are two particularly handy facts to have in your back pocket, especially on Data Sufficiency, precisely because they are true for every triangle under the Sun.
But just as there are many more people who don’t live in the Hamptons than do, just as there are many more former Major League Baseball players who aren’t in the Baseball Hall of Fame then those who are, so there are many more non-right triangles than right triangles. This is a post about them, about the 99% of triangles. What’s true, not just for elite triangle, but for all triangles?
The One Everyone Knows
Well, first of all, the sum of the three angles of any triangle is 180º. Everyone knows that already. A couple cool consequences:
(1) if all three angles are equal (an equiangular, equilateral triangle), then all three angles must be 180º/3 = 60º;
(2) in a right triangle, the sum of the two acute angles must be 90º.
Both are good fact to have at your fingertips on the GMAT.But, there we go, with the elite folks again. Back to everyone else.
The Triangle Inequality
That has a scary sounding name, doesn’t it? It sounds really sophisticated. In fact, it’s an idea so simple that a small child could understand the basics. Formally, it says: the sum of any two sides of a triangle must be greater than the third. In picture form:
Attachment:
11.JPG [ 17.77 KiB | Viewed 7451 times ]
The Triangle Inequality produces these three inequalities for any triangle. Now, think about why this has to be true. The shortest distance between point A and point C has to be the straight line: this is the piece where we’d expect a little kid to understand. If we take a “crooked” path from A to C (via point B), that has to be longer than taking the straight line distance, represented by AC. That’s the simple idea at the heart of the Triangle Inequality.
The Side-Angle Inequalities
Just as a political discussion of “the 99%” inevitably involves issues of socioeconomic inequality, so too, even when we leave the elite triangles and wander through the great unwashed masses of ordinary triangles, we run into geometric inequalities. This is another.
The Side Angle Inequalities. First, the technical way to say it: the measures of the three angles of a triangle are unequal in the same order as the lengths of theiropposite sides. That’s difficult to understand. Next, a picture:
Attachment:
12.JPG [ 17.14 KiB | Viewed 7422 times ]
Now, the really down-to-earth, straightforward way to say the idea is: the biggest side is always opposite the biggest angle, and the smallest side is always opposite the smallest angle. (In fact, they only reason I mentioned those other complicated ways to say it was to give you the challenge of figuring out why they just say the same thing as the simple bold statement.)
True For All
Those two mathematical patterns, the Triangle Inequality and the Side-Angle Inequalities, are true for all triangles — both the elite and not-elite triangles. On GRE Math, these are two particularly handy facts to have in your back pocket, especially on Data Sufficiency, precisely because they are true for every triangle under the Sun.
Director

Joined: 16 May 2014
Posts: 592
Given Kudos: 0
GRE 1: Q165 V161
Re: Facts about Ordinary Triangles on the GRE
[#permalink]
 15 Sep 2014, 14:19
15 Sep 2014, 14:19
Expert Reply
Example Problem
Two sides of a triangle have length 6 and 8. Which of the following are possible areas of the triangle?
I. 2
II. 12
III. 24
A) I only
B) I and II only
C) II and III only
D) I and III only
E) I, II, and III
Show: :: Answer
E







