Definition

A line forming a closed loop, every point on which is a fixed distance from a center point. Circle could also be defined as the set of all points equidistant from the center.




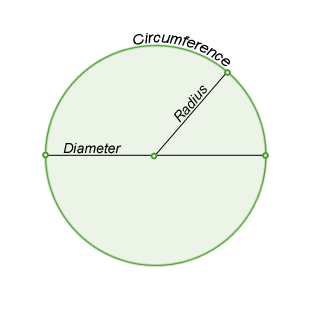
Center -a point inside the circle. All points on the circle are equidistant (same distance) from the center point.

Radius - the distance from the center to any point on the circle. It is half the diameter.

Diameter -t he distance across the circle. The length of any chord passing through the center. It is twice the radius.

Circumference - the distance around the circle.

Area - strictly speaking a circle is a line, and so has no area. What is usually meant is the area of the region enclosed by the circle.

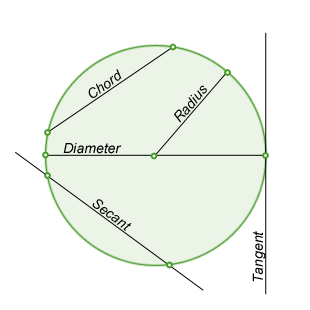
Chord - line segment linking any two points on a circle.

Tangent -a line passing a circle and touching it at just one point.
The tangent line is always at the 90 degree angle (perpendicular) to the radius of a circle.

Secant A line that intersects a circle at two points.

\(\pi\) In any circle, if you divide the circumference (distance around the circle) by it's diameter (distance across the circle), you always get the same number. This number is called Pi and is approximately 3.142.




• A circle is the shape with the largest area for a given length of perimeter (has the highest area to length ratio when compared to other geometric figures such as triangles or rectangles)
• All circles are similar
• To form a unique circle, it needs to have 3 points which are not on the same line.

Circumference, Perimeter of a circle

Given a radius \(r\) of a circle, the circumference can be calculated using the formula: \(Circumference=2\pi{r}\)

If you know the diameter \(D\) of a circle, the circumference can be found using the formula: \(Circumference=\pi{D}\)

If you know the area \(A\) of a circle, the circumference can be found using the formula: \(Circumference=\sqrt{4\pi{A}}\)


Area enclosed by a circle

Given the radius \(r\) of a circle, the area can be calculated using the formula: \(Area={\pi}r^2\)

If you know the diameter \(D\) of a circle, the area can be found using the formula: \(Area=\frac{{\pi}D^2}{4}\)

If you know the circumference \(C\) of a circle, the area can be found using the formula: \(Area=\frac{C^2}{4{\pi}}\)


Semicircle

Half a circle. A closed shape consisting of half a circle and a diameter of that circle.




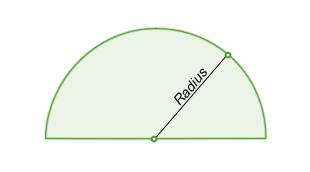
• The area of a semicircle is half the area of the circle from which it is made: \(Area=\frac{{\pi}r^2}{2}\)

• The perimeter of a semicircle is not half the perimeter of a circle. From the figure above, you can see that the perimeter is the curved part, which is half the circle, plus the diameter line across the bottom. So, the formula for the perimeter of a semicircle is: \(Perimeter=\pi{r}+2r=r(\pi+2)\)

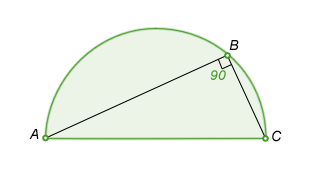
• The angle inscribed in a semicircle is always 90°.

• Any diameter of a circle subtends a right angle to any point on the circle. No matter where the point is, the triangle formed with diameter is always a right triangle.



Chord


A line that links two points on a circle or curve.




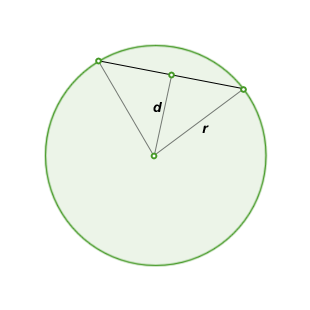
• A diameter is a chord that contains the center of the circle.
• Below is a formula for the length of a chord if you know the radius and the perpendicular distance from the chord to the circle center. This is a simple application of Pythagoras' Theorem.
\(Length=2\sqrt{r^2-d^2}\), where \(r\) is the radius of the circle, \(d\) is the perpendicular distance from the chord to the circle center.
• In a circle, a radius perpendicular to a chord bisects the chord. Converse: In a circle, a radius that bisects a chord is perpendicular to the chord, or In a circle, the perpendicular bisector of a chord passes through the center of the circle.


Angles in a circle

An inscribed angle is an angle ABC formed by points A, B, and C on the circle's circumference.




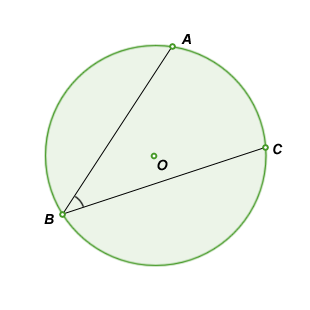
• Given two points A and C, lines from them to a third point B form the inscribed angle ∠ABC. Notice that the inscribed angle is constant. It only depends on the position of A and C.
• If you know the length \(L\) of the minor arc and radius, the inscribed angle is: \(Angle=\frac{90L}{\pi{r}}\)

A central angle is an angle AOC with endpoints A and C located on a circle's circumference and vertex O located at the circle's center. A central angle in a circle determines an arc AC.




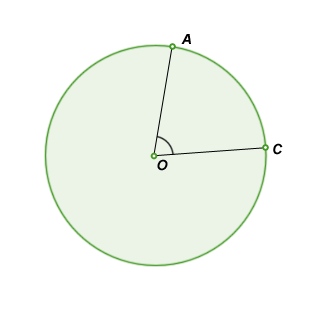
• The Central Angle Theorem states that the measure of inscribed angle is always half the measure of the central angle.




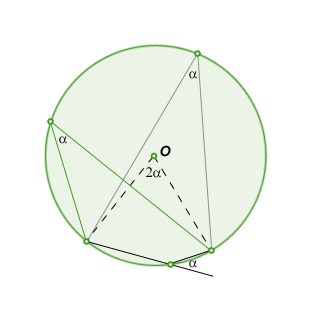
• An inscribed angle is exactly half the corresponding central angle. Hence, all inscribed angles that subtend the same arc are equal. Angles inscribed on the arc are supplementary. In particular, every inscribed angle that subtends a diameter is a right angle (since the central angle is 180 degrees).


Arcs and Sectors

A portion of the circumference of a circle.




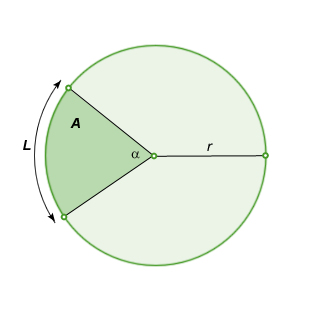
• Major and Minor Arcs Given two points on a circle, the minor arc is the shortest arc linking them. The major arc is the longest. On the GMAT, we usually assume the minor (shortest) arc.

• Arc Length The formula the arc measure is: \(L=2\pi{r}\frac{C}{360}\), where C is the central angle of the arc in degrees. Recall that \(2\pi{r}\) is the circumference of the whole circle, so the formula simply reduces this by the ratio of the arc angle to a full angle (360). By transposing the above formula, you solve for the radius, central angle, or arc length if you know any two of them.

• Sector is the area enclosed by two radii of a circle and their intercepted arc. A pie-shaped part of a circle.

• Area of a sector is given by the formula: \(Area=\pi{r^2}\frac{C}{360}\), where: C is the central angle in degrees. What this formula is doing is taking the area of the whole circle, and then taking a fraction of that depending on the central angle of the sector. So for example, if the central angle was 90°, then the sector would have an area equal to one quarter of the whole circle.


Power of a Point Theorem

Given circle O, point P not on the circle, and a line through P intersecting the circle in two points. The product of the length from P to the first point of intersection and the length from P to the second point of intersection is constant for any choice of a line through P that intersects the circle. This constant is called the "power of point P".

If P is outside the circle:




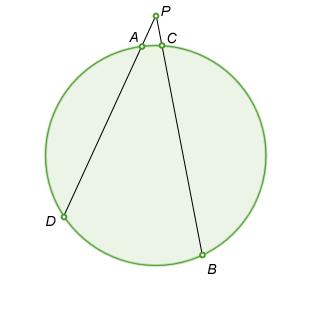
\(PA*PD=PC*PB=Constant\) - This becomes the theorem we know as the theorem of intersecting secants.

If P is inside the circle:




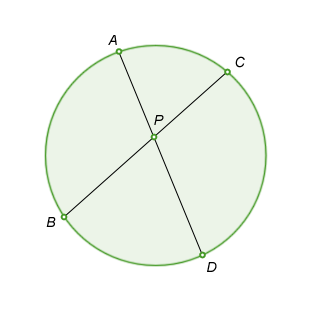
\(PA*PD=PC*PB=Constant\) - This becomes the theorem we know as the theorem of intersecting chords.

Tangent-Secant




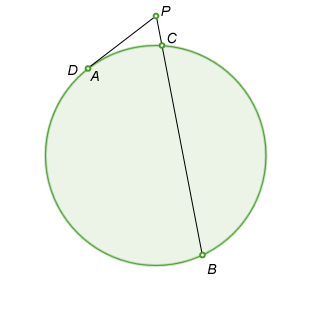
Should one of the lines be tangent to the circle, point A will coincide with point D, and the theorem still applies:

\(PA*PD=PC*PB=Constant\)

\(PA^2=PC*PB=Constant\) - This becomes the theorem we know as the theorem of secant-tangent theorem.

Two tangents




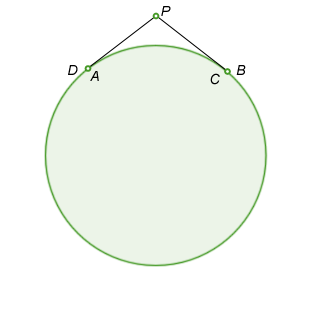
Should both of the lines be tangents to the circle, point A coincides with point D, point C coincides with point B, and the theorem still applies:

\(PA*PD=PC*PB=Constant\)

\(PA^2=PC^2\)

\(PA=PC\)