
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Manager

Joined: 10 Dec 2020
Posts: 50
Given Kudos: 955
GRE 1: Q166 V162
In the figure above, Lines m and n are straight, and the various angle
[#permalink]
 29 Nov 2021, 14:08
29 Nov 2021, 14:08
1
2
Bookmarks
Question Stats:
 90% (01:10) correct
90% (01:10) correct
 10% (03:05) wrong
10% (03:05) wrong  based on 10 sessions
based on 10 sessions
Hide Show timer Statistics

In the figure above, Lines m and n are straight, and the various angles have measures as shown. If x + y = 80 what is the value of z – y?
_______
Show: ::
100
Manager

Joined: 10 Dec 2020
Posts: 50
Given Kudos: 955
GRE 1: Q166 V162
Re: In the figure above, Lines m and n are straight, and the various angle
[#permalink]
 29 Nov 2021, 14:10
29 Nov 2021, 14:10
Official explanation by Manhattan GRE:
Because n is a straight line, the 4 angles above line n must add up to 180 degrees:
x + 3y = 180
We are also told that x + y = 80. The easiest method for solving these two equations involving two unknowns x and y is to simply subtract the second equation from the first, eliminating x in the process:
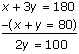
Therefore y = 50. Next, using x + y = 80 we obtain x = 30. Finally, noting that angles x and z together constitute the “underside” of straight line m, we can write:
x + z = 180.
This leads us to z = 150. The final answer to the question is given by:
z – y = 150 – 50 = 100.
Of course, we could also have observed that the angle with measure z degrees is opposite the totality of the 3 angles with measure y degrees each, such that z = 3 y . This would have made it unnecessary to solve for x as an intermediate step. Furthermore, knowing z = 3 y we could then write:
z – y = 3y – y = 2y.
Thus we could obtain the answer directly as 2y = 100, as derived above.
The correct answer is 100.
Because n is a straight line, the 4 angles above line n must add up to 180 degrees:
x + 3y = 180
We are also told that x + y = 80. The easiest method for solving these two equations involving two unknowns x and y is to simply subtract the second equation from the first, eliminating x in the process:
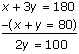
Therefore y = 50. Next, using x + y = 80 we obtain x = 30. Finally, noting that angles x and z together constitute the “underside” of straight line m, we can write:
x + z = 180.
This leads us to z = 150. The final answer to the question is given by:
z – y = 150 – 50 = 100.
Of course, we could also have observed that the angle with measure z degrees is opposite the totality of the 3 angles with measure y degrees each, such that z = 3 y . This would have made it unnecessary to solve for x as an intermediate step. Furthermore, knowing z = 3 y we could then write:
z – y = 3y – y = 2y.
Thus we could obtain the answer directly as 2y = 100, as derived above.
The correct answer is 100.
Re: In the figure above, Lines m and n are straight, and the various angle
[#permalink]
 16 Sep 2024, 14:00
16 Sep 2024, 14:00
1
XavierAlexander wrote:

In the figure above, Lines m and n are straight, and the various angles have measures as shown. If x + y = 80 what is the value of z – y?
Since z and x form straight line m, z+x=180.
Subtracting x+y=80 from z+x=180, we get:
(z+x) - (x+y) = 180-80
z-y = 100




