
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
All you need to know about Quantitative Comparisons
[#permalink]
 09 Jan 2017, 22:52
09 Jan 2017, 22:52
4
1
Bookmarks
Quantitative Comparison (QC) questions are unique to the GRE. They may seem a little strange at first, but once mastered will get you well on your way to a great quant score.
So what exactly are quantitative comparisons and what do they test?
Quantitative Comparison questions require you to make a comparison between quantities in two columns. They follow a consistent pattern. You are presented with two quantities — Quantity A and Quantity B and four answer choices that never change. The four answer choices are:
(A) Quantity A is greater
(B) Quantity B is greater
(C) The two quantities are equal
(D) The relationship cannot be determined from the information given.
You are to decide if one column is ALWAYS greater, if the columns are ALWAYS equal, or if no comparison can be determined from the information given. The first step to conquering QCs is memorizing what these answer choices mean so that you don’t have to spend precious time reading them come test day.
Quantitative Comparisons test your ability to break down pieces of information, to make smart approximations, to perform simple math calculations and to use common sense to quickly compare two given quantities.
Strategies to solve Quantitative Comparisons
The hardest part of answering a QC question is knowing where to begin. You can save considerable time and frustration if you develop good habits now that carry over to the exam later. So here are some strategies that will definitely come in handy come test day.
1.Compare. Don’t calculate…… Approximate if necessary:
The first important thing to remember is that you don’t always have to actually do the math. Look for ways to simplify the comparison without wasting time on a lot of arithmetic. Let’s say you’re presented with the following question

Capture 1.PNG [ 1.52 KiB | Viewed 5657 times ]
Quantity A has an even exponent, so it will have to be a positive number. Quantity B has an odd exponent, so it will have to be a negative number. That’s enough to answer the question, so pick option A.
Sometimes you will need to make smart approximations and then compare the two quantities.
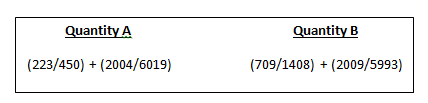
Capture 2.PNG [ 2.49 KiB | Viewed 5667 times ]
223/450 is slightly lesser than ½ (since 225/450 = ½), 2004/6019 is slightly lesser than 1/3 (since 2004/6012 = 1/3), 709/1408 is slightly greater than ½ (since 709/1418 = ½) and 2009/5993 is slightly greater than 1/3 (since 2009/6027 = 1/3). The question now can be rewritten as

Capture 3.PNG [ 4.79 KiB | Viewed 5624 times ]
2.Plugging in Values:
Whenever you have variables as quantities, plug in values. When plugging in values keep in mind the following two points :
a. Plug in values and try to disprove the answer choices A, B and C (try proving the answer to be D). If you plug in a value which makes both the columns equal, then try to plug in another value which makes one column greater.
b. Plug in numbers 0, 1, 2, –1, –2, and 1⁄2, specifically in that order. These numbers cover most of the contingencies: positive, negative, zero, odd, even, fraction.
Let us look at the example given below

Capture 4.PNG [ 1.29 KiB | Viewed 5646 times ]
If x = 0, then the two quantities are alike, and C prevails. But if x = 2, then Quantity A = 4 and Quantity B = 16, and the answer is B. Therefore, the answer can be C or B, depending on what you plug in. A definite relationship cannot be determined. Pick answer option D.
Notice how we did not plug in x = 1 (since this again would have made the two quantities alike) but instead plugged in x = 2 which gave us a different relationship between the two quantities than when we plugged in x = 0.
3. Simplifying the comparison:
If both quantities are algebraic or arithmetic expressions and you cannot easily see a relationship between them, you can try to simplify the comparison. The following are some rules that you have to adhere to while simplifying comparisons:
a. You can add or subtract both quantities by the same value
b. You can multiply or divide both quantities by a positive value
c. Squaring both columns is permissible, as long as each side is always positive
d. You cannot multiply or divide both quantities by a negative number
e. You cannot multiply or divide both quantities with a variable if the sign of the variable is unknown
Let’s say that you are presented with the following question. Instead of plugging in values we can simplify the expressions as follows.

Capture 5.PNG [ 6.62 KiB | Viewed 5649 times ]
Here is another example
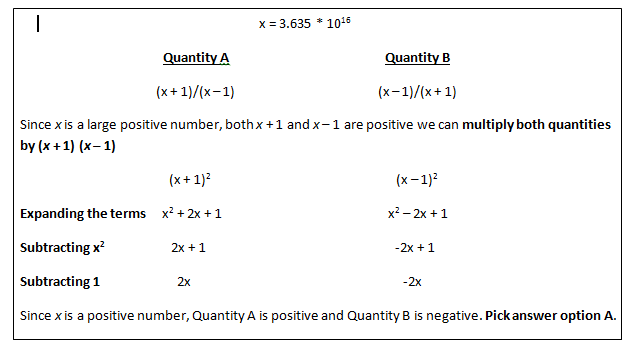
Capture 6.PNG [ 11.52 KiB | Viewed 5670 times ]
4. Geometric figures are not necessarily drawn to scale:
When you see a diagram in a problem solving question, you can generally assume that it’s drawn to scale. However, the opposite is true for QCs. Never make any assumptions based on the diagram given in a QC question, hold onto only definite pieces of information. For example, an angle that looks like a right angle may in fact have a value of 30° when you work out the math.
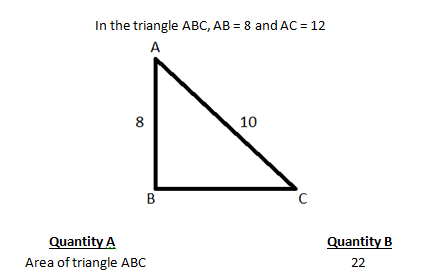
Capture 7.PNG [ 8.16 KiB | Viewed 5651 times ]
The angle at B appears to be a right angle, but absolutely nothing in the diagram or the text given guarantees that B is a right angle.
On the left, the triangle has been crushed close to flat, and it could be crushed even further, to an area of almost zero. Certainty the area could be less than 22. By contrast, if AB and BC are perpendicular, then the area would be A = (½)bh = (½)(8)(6) = 24, which is more than 22. Depending on the diagram, it could go either way. Pick answer option D.
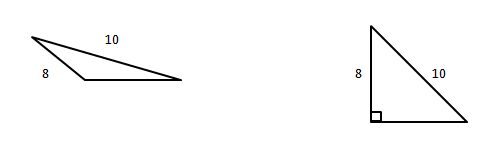
Capture 8.PNG [ 2.55 KiB | Viewed 5652 times ]
The above strategies should help you proceed without reservation or hesitation when you encounter a seemingly ugly Quantitative Comparisons. Remember that these questions when approached in the right way are time savers because you are not required to calculate, but compare.
Do leave comments / feedback if this article helped you.
Cheers!
CrackVerbal Academics Team
So what exactly are quantitative comparisons and what do they test?
Quantitative Comparison questions require you to make a comparison between quantities in two columns. They follow a consistent pattern. You are presented with two quantities — Quantity A and Quantity B and four answer choices that never change. The four answer choices are:
(A) Quantity A is greater
(B) Quantity B is greater
(C) The two quantities are equal
(D) The relationship cannot be determined from the information given.
You are to decide if one column is ALWAYS greater, if the columns are ALWAYS equal, or if no comparison can be determined from the information given. The first step to conquering QCs is memorizing what these answer choices mean so that you don’t have to spend precious time reading them come test day.
Quantitative Comparisons test your ability to break down pieces of information, to make smart approximations, to perform simple math calculations and to use common sense to quickly compare two given quantities.
Strategies to solve Quantitative Comparisons
The hardest part of answering a QC question is knowing where to begin. You can save considerable time and frustration if you develop good habits now that carry over to the exam later. So here are some strategies that will definitely come in handy come test day.
1.Compare. Don’t calculate…… Approximate if necessary:
The first important thing to remember is that you don’t always have to actually do the math. Look for ways to simplify the comparison without wasting time on a lot of arithmetic. Let’s say you’re presented with the following question
Attachment:
Capture 1.PNG [ 1.52 KiB | Viewed 5657 times ]
Quantity A has an even exponent, so it will have to be a positive number. Quantity B has an odd exponent, so it will have to be a negative number. That’s enough to answer the question, so pick option A.
Sometimes you will need to make smart approximations and then compare the two quantities.
Attachment:
Capture 2.PNG [ 2.49 KiB | Viewed 5667 times ]
223/450 is slightly lesser than ½ (since 225/450 = ½), 2004/6019 is slightly lesser than 1/3 (since 2004/6012 = 1/3), 709/1408 is slightly greater than ½ (since 709/1418 = ½) and 2009/5993 is slightly greater than 1/3 (since 2009/6027 = 1/3). The question now can be rewritten as
Attachment:
Capture 3.PNG [ 4.79 KiB | Viewed 5624 times ]
2.Plugging in Values:
Whenever you have variables as quantities, plug in values. When plugging in values keep in mind the following two points :
a. Plug in values and try to disprove the answer choices A, B and C (try proving the answer to be D). If you plug in a value which makes both the columns equal, then try to plug in another value which makes one column greater.
b. Plug in numbers 0, 1, 2, –1, –2, and 1⁄2, specifically in that order. These numbers cover most of the contingencies: positive, negative, zero, odd, even, fraction.
Let us look at the example given below
Attachment:
Capture 4.PNG [ 1.29 KiB | Viewed 5646 times ]
If x = 0, then the two quantities are alike, and C prevails. But if x = 2, then Quantity A = 4 and Quantity B = 16, and the answer is B. Therefore, the answer can be C or B, depending on what you plug in. A definite relationship cannot be determined. Pick answer option D.
Notice how we did not plug in x = 1 (since this again would have made the two quantities alike) but instead plugged in x = 2 which gave us a different relationship between the two quantities than when we plugged in x = 0.
3. Simplifying the comparison:
If both quantities are algebraic or arithmetic expressions and you cannot easily see a relationship between them, you can try to simplify the comparison. The following are some rules that you have to adhere to while simplifying comparisons:
a. You can add or subtract both quantities by the same value
b. You can multiply or divide both quantities by a positive value
c. Squaring both columns is permissible, as long as each side is always positive
d. You cannot multiply or divide both quantities by a negative number
e. You cannot multiply or divide both quantities with a variable if the sign of the variable is unknown
Let’s say that you are presented with the following question. Instead of plugging in values we can simplify the expressions as follows.
Attachment:
Capture 5.PNG [ 6.62 KiB | Viewed 5649 times ]
Here is another example
Attachment:
Capture 6.PNG [ 11.52 KiB | Viewed 5670 times ]
4. Geometric figures are not necessarily drawn to scale:
When you see a diagram in a problem solving question, you can generally assume that it’s drawn to scale. However, the opposite is true for QCs. Never make any assumptions based on the diagram given in a QC question, hold onto only definite pieces of information. For example, an angle that looks like a right angle may in fact have a value of 30° when you work out the math.
Attachment:
Capture 7.PNG [ 8.16 KiB | Viewed 5651 times ]
The angle at B appears to be a right angle, but absolutely nothing in the diagram or the text given guarantees that B is a right angle.
On the left, the triangle has been crushed close to flat, and it could be crushed even further, to an area of almost zero. Certainty the area could be less than 22. By contrast, if AB and BC are perpendicular, then the area would be A = (½)bh = (½)(8)(6) = 24, which is more than 22. Depending on the diagram, it could go either way. Pick answer option D.
Attachment:
Capture 8.PNG [ 2.55 KiB | Viewed 5652 times ]
The above strategies should help you proceed without reservation or hesitation when you encounter a seemingly ugly Quantitative Comparisons. Remember that these questions when approached in the right way are time savers because you are not required to calculate, but compare.
Do leave comments / feedback if this article helped you.
Cheers!
CrackVerbal Academics Team
Re: All you need to know about Quantitative Comparisons
[#permalink]
 24 Jan 2017, 12:42
24 Jan 2017, 12:42
Thanks a lot! Love these type of articles. Very helpful.

gmatclubot
Re: All you need to know about Quantitative Comparisons [#permalink]
24 Jan 2017, 12:42
Moderators:





