GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
In triangle PQR, the measure of angle P is 30° greater than twice the
[#permalink]
22 Feb 2024, 01:49
22 Feb 2024, 01:49
Expert Reply
00:00
Question Stats:
100% (01:24) correct
0% (00:00) wrong based on 5 sessions
Hide Show timer Statistics
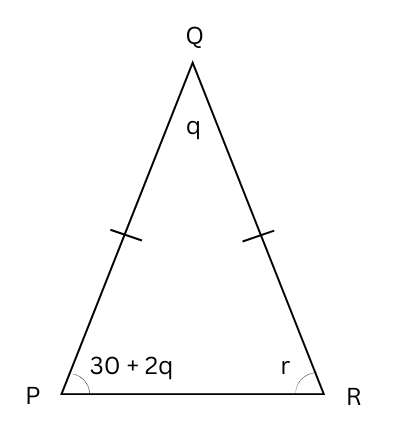
In triangle PQR, the measure of angle P is 30° greater than twice the measure of angle Q. If PQ = QR, what is the measure of angle R?
Show: ::
78
Part of the project: QOTD - Question of the day!!! - Gain 20 Kudos & Get FREE Access to GRE Prep Club TESTS
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Free Materials for the GRE General Exam - Where to get it!!
Shorter GRE - PREPARATION STRATEGY
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Free Materials for the GRE General Exam - Where to get it!!
Shorter GRE - PREPARATION STRATEGY
Manager
Joined: 11 Nov 2023
Posts: 207
Given Kudos: 76
WE:Business Development (Advertising and PR)
Re: In triangle PQR, the measure of angle P is 30° greater than twice the
[#permalink]
22 Feb 2024, 12:05
22 Feb 2024, 12:05
1
We're given that 2 sides of the triangle are equal, but one of its angles, Q, is different from the other angles. Therefore, PQR is an isosceles triangle and the measures of angles P and R are equal. (The two angles opposite from the two equal sides of an isosceles triangle are equal.)
Since the measure of angle P is 30° greater than twice the measure of angle Q:
P = 30+2Q = R
The interior angles of a triangle always add up to 180°:
P + Q + R = 180
(30+2Q) + Q + (30+2Q) = 180
5Q + 60 = 180
Q = 24
Solve for R:
30 + 2(Q) = 30 + 2(24) = 78
trianglePQR.png [ 19.02 KiB | Viewed 590 times ]
Since the measure of angle P is 30° greater than twice the measure of angle Q:
P = 30+2Q = R
The interior angles of a triangle always add up to 180°:
P + Q + R = 180
(30+2Q) + Q + (30+2Q) = 180
5Q + 60 = 180
Q = 24
Solve for R:
30 + 2(Q) = 30 + 2(24) = 78
Attachment:
trianglePQR.png [ 19.02 KiB | Viewed 590 times ]
gmatclubot
Re: In triangle PQR, the measure of angle P is 30° greater than twice the [#permalink]
22 Feb 2024, 12:05
Moderators:
