
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Director

Joined: 16 May 2014
Posts: 592
Given Kudos: 0
GRE 1: Q165 V161
Practice Q#5- Coordinate Geometry Circle
[#permalink]
 03 Aug 2014, 11:00
03 Aug 2014, 11:00
Expert Reply
Question Stats:
 75% (02:26) correct
75% (02:26) correct
 24% (02:04) wrong
24% (02:04) wrong  based on 33 sessions
based on 33 sessions
Hide Show timer Statistics
Try this tricky Geometry question

math1.JPG [ 28.8 KiB | Viewed 6282 times ]
Attachment:
math1.JPG [ 28.8 KiB | Viewed 6282 times ]
Re: Practice Q#5
[#permalink]
 04 Aug 2014, 17:30
04 Aug 2014, 17:30
3
Lets name the point of intersection of the perpendicular from OQ on the line OR as X.
Thus forming a triangle named OQX. Now, from the figure angle QOR = 180-150=30 degrees, this makes triangle OQX a 30-60-90 triangle with OQ=4. From this, we know that the sides of the triangles are in a ratio of \(\sqrt{3}\):2:1, hence in triangle OQX, OQ = 4,\(OX = 2\sqrt{3}\), QX=2.
As angle QOR = 30 degree, the length of the arc \(QR = (30/360) X 2pi X OQ\) = \(2pi/3\).
As per, the question the perimeter of the shaded region would be,
length of QX + length of XR + length of arc QR = \(2 + (4 - 2\sqrt{3}) + 2pi/3\) = \(2pi/3 - 2\sqrt{3} + 6\)
Hence, the correct answer would be (D)
Thus forming a triangle named OQX. Now, from the figure angle QOR = 180-150=30 degrees, this makes triangle OQX a 30-60-90 triangle with OQ=4. From this, we know that the sides of the triangles are in a ratio of \(\sqrt{3}\):2:1, hence in triangle OQX, OQ = 4,\(OX = 2\sqrt{3}\), QX=2.
As angle QOR = 30 degree, the length of the arc \(QR = (30/360) X 2pi X OQ\) = \(2pi/3\).
As per, the question the perimeter of the shaded region would be,
length of QX + length of XR + length of arc QR = \(2 + (4 - 2\sqrt{3}) + 2pi/3\) = \(2pi/3 - 2\sqrt{3} + 6\)
Hence, the correct answer would be (D)
Practice Q#5- Coordinate Geometry Circle
[#permalink]
 04 Jan 2024, 01:48
04 Jan 2024, 01:48
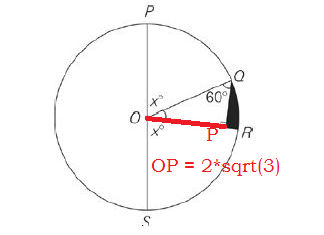
Let us call the point where a line from Q drops on to the line OR at an angle of 90 degrees as P. We have to find the perimeter of the figure PQO.
We now have a triangle PQO, which by the given measures is a 30-60-90 triangle.
Now the sides of a 30-60-90 triangle are in the ratio \(x:\sqrt{3}x:2x\) (short side:long side:hypotenuse)
Now we know that the hypotenuse OQ is nothing but the radius and is thus equal to 4 and is equal to 2x in the above ratio. This means that the value of x is 2. From this the we know that the value of the long side OP is \(\sqrt{3}*2 = 2*\sqrt{3}\). And the length of the short side PQ is x, which is equal to 2.
OQ= 4
OP = \(2\sqrt{3}\)
perimeter of shaded region - 2.4.png [ 20.16 KiB | Viewed 1546 times ]
PQ = 2

perimeter of shaded region - 2.1.png [ 19.44 KiB | Viewed 1550 times ]
Now we the know value of one side of the shaded region - PQ = 2.
Now to calculate the value of PR.
From the figure, PR = OR - OP
OR = 4 (the radius)
OP = \(2\sqrt{3}\) (the long side of triangle PQO)
PR = OR - OP
PR = \(4 - 2\sqrt{3}\)

perimeter of shaded region - 2.2.png [ 20.44 KiB | Viewed 1540 times ]
Now we know the value of one more side - PR - of the shaded region PQR.
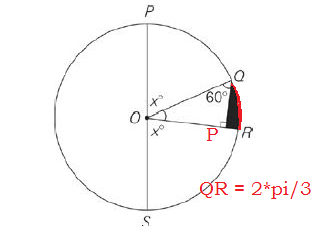
Now we need to know the value of the third side of the shaded region - the curve QR.
This can be easily found out since we are given the diameter of the circle PS = 8. This means the radius is 4 and the circumference is \(2*\pi*4 = 8*\pi.\)
Now we want to know the value of the central angle QOR. We know this to be 30 degs since it is a part of 30-60-90 triangle.
Now the length of the Arc QR can be found out from the ratio
\(\dfrac{30}{360} = \dfrac{x}{8*\pi}\)
\(\dfrac{1}{12} = \dfrac{x}{8*\pi}\)
\(x = \dfrac{8*\pi}{12} \)
\(x = \dfrac{2*\pi}{3}\)
\(QR = \dfrac{2*\pi}{3}\)
perimeter of shaded region - 2.3.png [ 20.28 KiB | Viewed 1542 times ]
Now we can find the perimeter of the shaded region
PQR = PQ + PR + QR = \(2 + 4 - 2\sqrt{3} + \dfrac{2*\pi}{3} = \dfrac{2*\pi}{3} - 2\sqrt{3} + 6\)
The answer is Choice D - \(\dfrac{2*\pi}{3} - 2\sqrt{3} + 6\)
Attachment:
File comment: Drop point P from Q
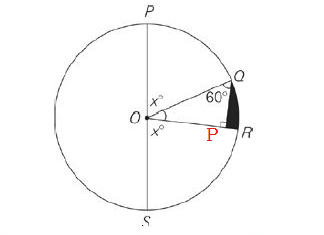
perimeter of shaded region - 2.png [ 18.86 KiB | Viewed 1538 times ]
perimeter of shaded region - 2.png [ 18.86 KiB | Viewed 1538 times ]
We now have a triangle PQO, which by the given measures is a 30-60-90 triangle.
Now the sides of a 30-60-90 triangle are in the ratio \(x:\sqrt{3}x:2x\) (short side:long side:hypotenuse)
Now we know that the hypotenuse OQ is nothing but the radius and is thus equal to 4 and is equal to 2x in the above ratio. This means that the value of x is 2. From this the we know that the value of the long side OP is \(\sqrt{3}*2 = 2*\sqrt{3}\). And the length of the short side PQ is x, which is equal to 2.
OQ= 4
OP = \(2\sqrt{3}\)
Attachment:
perimeter of shaded region - 2.4.png [ 20.16 KiB | Viewed 1546 times ]
PQ = 2
Attachment:
perimeter of shaded region - 2.1.png [ 19.44 KiB | Viewed 1550 times ]
Now we the know value of one side of the shaded region - PQ = 2.
Now to calculate the value of PR.
From the figure, PR = OR - OP
OR = 4 (the radius)
OP = \(2\sqrt{3}\) (the long side of triangle PQO)
PR = OR - OP
PR = \(4 - 2\sqrt{3}\)
Attachment:
perimeter of shaded region - 2.2.png [ 20.44 KiB | Viewed 1540 times ]
Now we know the value of one more side - PR - of the shaded region PQR.
Now we need to know the value of the third side of the shaded region - the curve QR.
This can be easily found out since we are given the diameter of the circle PS = 8. This means the radius is 4 and the circumference is \(2*\pi*4 = 8*\pi.\)
Now we want to know the value of the central angle QOR. We know this to be 30 degs since it is a part of 30-60-90 triangle.
Now the length of the Arc QR can be found out from the ratio
\(\dfrac{30}{360} = \dfrac{x}{8*\pi}\)
\(\dfrac{1}{12} = \dfrac{x}{8*\pi}\)
\(x = \dfrac{8*\pi}{12} \)
\(x = \dfrac{2*\pi}{3}\)
\(QR = \dfrac{2*\pi}{3}\)
Attachment:
perimeter of shaded region - 2.3.png [ 20.28 KiB | Viewed 1542 times ]
Now we can find the perimeter of the shaded region
PQR = PQ + PR + QR = \(2 + 4 - 2\sqrt{3} + \dfrac{2*\pi}{3} = \dfrac{2*\pi}{3} - 2\sqrt{3} + 6\)
The answer is Choice D - \(\dfrac{2*\pi}{3} - 2\sqrt{3} + 6\)





