
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
A species of insect has an average mass of 5.2 grams and a s
[#permalink]
 28 Jul 2018, 16:28
28 Jul 2018, 16:28
2
Expert Reply
7
Bookmarks
Question Stats:
 49% (01:15) correct
49% (01:15) correct
 50% (01:44) wrong
50% (01:44) wrong  based on 125 sessions
based on 125 sessions
Hide Show timer Statistics
A species of insect has an average mass of 5.2 grams and a standard deviation of 0.6 grams. The mass of the insects follows a normal distribution.
A) Quantity A is greater.
B) Quantity B is greater.
C) The two quantities are equal.
D) The relationship cannot be determined from the information given.
Quantity A |
Quantity B |
The percent of the insects that have a mass between 5.2 and 5.8 grams |
The percent of the insects that have a mass between 4.9 and 5.5 grams |
A) Quantity A is greater.
B) Quantity B is greater.
C) The two quantities are equal.
D) The relationship cannot be determined from the information given.
Re: A species of insect has an average mass of 5.2 grams and a s
[#permalink]
 01 Sep 2018, 10:35
01 Sep 2018, 10:35
7
Expert Reply
1
Bookmarks
yashkanoongo wrote:
I chose C. I am not very clear with the explanation in support of B. Since 4.9 - 5.5 lies exactly between -1 SD and +1 SD isn't the % of insects with this mass also 34%? So shouldn't the percentages be equal, at least mathematically?
Not exactly: Notice quantity A is one standard deviation from mean i.e. mean is the lowest value. But quantity B is centered around mean.
Qty is 34.1% where as Qty B is 38.2% to be exact. This is best shown in image below!
Attachment:
Capture.PNG [ 28.99 KiB | Viewed 11391 times ]
General Discussion
Re: A species of insect has an average mass of 5.2 grams and a s
[#permalink]
 12 Aug 2018, 16:21
12 Aug 2018, 16:21
7
Expert Reply
Explanation
Whenever the words “Normal distribution” appear on the GRE, draw a bell-curve diagram that approximates the one below. Memorize the numbers 34 : 14 : 2.
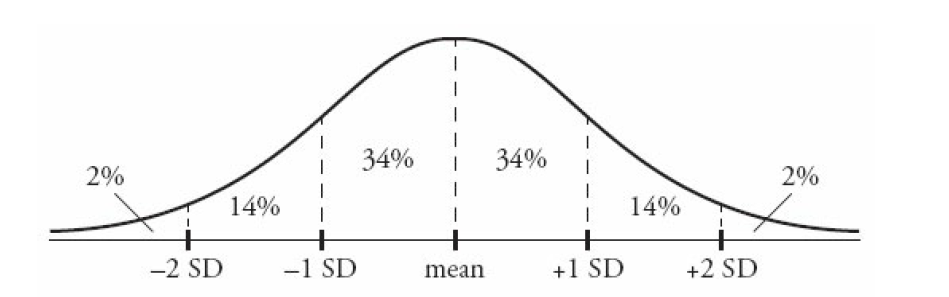
Capture.PNG [ 68.26 KiB | Viewed 11458 times ]
The middle of the bell curve is the average, or mean, so place 5.2 underneath the 0 in the center; 34%, 14%, and 2% represent the approximate percentages that fall between the standard deviation lines.
For instance, 14% of the population falls between 1 and 2 standard deviations below the mean. Now, use the standard deviation of 0.6 grams to figure out the exact dividing lines between the marked regions of the normal curve. The mass of an insect that is exactly 1 standard deviation above the mean is 5.2 + 0.6 = 5.8, and the mass of one that is 1 standard deviation below the mean is 5.2 – 0.6 = 4.6.
Similarly, the mass at exactly 2 standard deviations above the mean is 6.4 and at 2 below is 4.0.

Capture.PNG [ 82.33 KiB | Viewed 11457 times ]
Quantity A, the percent between 5.2 and 5.8 grams, is 34%.
However, Quantity B will require some estimating. Note that 4.9 is halfway between 4.6 and 5.2, while 5.5 is halfway between 5.2 and 5.8. Therefore, the area between 4.9 and 5.5, while still a range of 0.6, is under the bigger part of the bell curve in the center. Since the area under the center is bigger than the area between 0 and 1 standard deviations, the percentage of the area under the center must also be greater. Therefore, Quantity B is greater.
Whenever the words “Normal distribution” appear on the GRE, draw a bell-curve diagram that approximates the one below. Memorize the numbers 34 : 14 : 2.
Attachment:
Capture.PNG [ 68.26 KiB | Viewed 11458 times ]
The middle of the bell curve is the average, or mean, so place 5.2 underneath the 0 in the center; 34%, 14%, and 2% represent the approximate percentages that fall between the standard deviation lines.
For instance, 14% of the population falls between 1 and 2 standard deviations below the mean. Now, use the standard deviation of 0.6 grams to figure out the exact dividing lines between the marked regions of the normal curve. The mass of an insect that is exactly 1 standard deviation above the mean is 5.2 + 0.6 = 5.8, and the mass of one that is 1 standard deviation below the mean is 5.2 – 0.6 = 4.6.
Similarly, the mass at exactly 2 standard deviations above the mean is 6.4 and at 2 below is 4.0.
Attachment:
Capture.PNG [ 82.33 KiB | Viewed 11457 times ]
Quantity A, the percent between 5.2 and 5.8 grams, is 34%.
However, Quantity B will require some estimating. Note that 4.9 is halfway between 4.6 and 5.2, while 5.5 is halfway between 5.2 and 5.8. Therefore, the area between 4.9 and 5.5, while still a range of 0.6, is under the bigger part of the bell curve in the center. Since the area under the center is bigger than the area between 0 and 1 standard deviations, the percentage of the area under the center must also be greater. Therefore, Quantity B is greater.








