GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
How many liters of a 40% iodine solution need to be mixed with 35 lite
[#permalink]
19 May 2021, 05:05
19 May 2021, 05:05
Expert Reply
00:00
Question Stats:
85% (01:40) correct
15% (02:21) wrong based on 20 sessions
Hide Show timer Statistics
How many liters of a 40% iodine solution need to be mixed with 35 liters of a 20% iodine solution to create a 35% iodine solution?
A. 35
B. 49
C. 100
D. 105
E. 140
Kudos for the right answer and explanation
Question part of the project GRE Quantitative Reasoning Daily Challenge - (2021) EDITION
GRE - Math Book
A. 35
B. 49
C. 100
D. 105
E. 140
Kudos for the right answer and explanation
Question part of the project GRE Quantitative Reasoning Daily Challenge - (2021) EDITION
GRE - Math Book
Retired Moderator
Joined: 10 Apr 2015
Posts: 6218
Given Kudos: 136
Re: How many liters of a 40% iodine solution need to be mixed with 35 lite
[#permalink]
19 May 2021, 05:57
19 May 2021, 05:57
1
Carcass wrote:
How many liters of a 40% iodine solution need to be mixed with 35 liters of a 20% iodine solution to create a 35% iodine solution?
A. 35
B. 49
C. 100
D. 105
E. 140
Kudos for the right answer and explanation
Question part of the project GRE Quantitative Reasoning Daily Challenge - (2021) EDITION
GRE - Math Book
A. 35
B. 49
C. 100
D. 105
E. 140
Kudos for the right answer and explanation
Question part of the project GRE Quantitative Reasoning Daily Challenge - (2021) EDITION
GRE - Math Book
One approach is to sketch the solutions with their components separated.
Let's start with the 35 liters of 20% iodine solution:
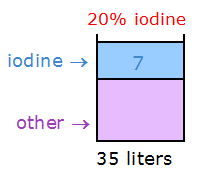
20% of 35 liters = 7 liters, so the original solution contains 7 liters of iodine.
To this solution, we'll add x liters of the solution that's 40% iodine.
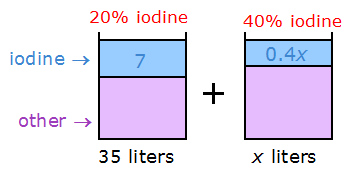
40% of x liters = 0.4x liters. So, we're adding 0.4x liters of iodine.
When we combine the two solutions, it's easy to see how the resulting mixture looks, since we need only add the volumes of iodine in each solution.
As you can see, our resulting mixture has a TOTAL volume of 35 + x liters.
We can also see that the resulting mixture contains 7 + 0.4x liters of iodine.
Since the resulting mixture must by 35% (aka 35/100) iodine, we can write the following equation:
(7 + 0.4x)/(35 + x liters) = 35/100
First simplify 35/100 to get: (7 + 0.4x)/(35 + x liters) = 7/20
Cross multiply to get: 20(7 + 0.4x) = 7(35 + x liters)
Expand: 140 + 8x = 245 + 7x
Solve: x = 105
Answer: D
gmatclubot
Re: How many liters of a 40% iodine solution need to be mixed with 35 lite [#permalink]
19 May 2021, 05:57
Moderators:
