
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
GRE Math Essentials - Equations
[#permalink]
 Updated on: 11 Apr 2023, 10:47
Updated on: 11 Apr 2023, 10:47
1
Expert Reply
1
Bookmarks
This is part of our GRE Math Essentials project & GRE Math Essentials - A most comprehensive handout!!! that are the best complement to our GRE Math Book. It provides a cutting-edge, in-depth overview of all the math concepts from basic to mid-upper levels. The book still remains our hallmark: from basic to the most advanced GRE math concepts tested during the exam. Moreover, the following chapters will give you many tips, tricks, and shortcuts to make your quant preparation more robust and solid.
Any form of numeric representation representing a number is called an Expression.
The equality of two expressions is called Equation.
1. \((a ± b)^2 = a^2 ± 2ab + b^2\)
2. \((a + b)^2 – (a – b)^2 = 4ab\)
3. \((a + b)^2 + (a – b)^2 = 2 (a^2+ b^2)\)
4. \(a^2 – b^2= (a + b) (a – b)\)
5.
(1) \((a + b)^3= a^3+ b^3+ 3ab (a + b)\);
(2) \((a – b)^3= a^3– b^3– 3ab (a – b)\)
6.
(1) \(a^3+ b^3= (a + b) (a^2– ab + b^2)\) ;
(2) \(a^3– b^3= (a – b) (a^2+ ab + b^2)\)
7. \((a + b + c)^2= a^2+ b^2+ c^2+ 2ab + 2bc + 2ac\)
8. \(a^3+ b^3+ c^3– 3abc = (a+b+c)(a^2+ b^2+ c^2– ab – ac – bc) ⇒ if (a + b + c) = 0\) then \(a^3+ b + c^3= 3abc.\)
Solutions for systems of equations
1. Definition of a solution to a statement of equation. A solution of a system of equations consists of values for the variables that reduce each equation of the system to a true statement. To solve a system of equations means to find all solutions to the system.
2. Consistent systems. When a system of equations has at least one solution, it is said to be consistent.
3. Unique solutions. When a system of equations has one and only one solution, we say that the solution is unique.
4. Possible sets of solutions for a system of equations. For a given system of equations.
(a). We may have one and only one solution. In this case we say we have a unique solution.
(b). We may no solutions. In this case we say that the system of equations is inconsistent.
5. We may have more than one set of solutions but the set of solutions is not infinite. We say that the number of solutions is finite. This can occur when one of more variables appears in an equation with a power other than one and/or there is a product of variables in one of the equations. Such an equation is called a non-linear equation.
6. We have an infinite number of solutions to the system of equations. In this case we say the system is indeterminate.
Methods of finding solutions for equations
Method of substitution.
(a): Pick one of the equations and solve for one of the variables in terms of the remaining variables.
(b): Substitute the result in the remaining equations.
(c): If one equation in one variable results, solve this equation. Otherwise, repeat step a until a single equation with a single variable remains.
(d): Find the values of the remaining variables by back-substitution.
(e): Check the solution found.
Examples of the method of substitution.
\(2x + 3y = 7 \)
\(x − y = − 1\)
\(x = y − 1\)
\(2(y − 1) + 3 y = 7\)
\(⇒ 2 y − 2+3 y = 7\)
\(⇒ 5 y = 9\)
\(⇒ y =\frac{9}{5}\)
\(x − \frac{9}{5} = − 1\)
\(⇒ x = − 1 + \frac{9}{5} = \frac{4}{5}\)
Method of elimination
1. Basic idea. The idea behind the method of elimination is to keep replacing the original equations in the system with equivalent equations until an obvious solution is reached. In effect we keep replacing equations until each equation contains just one variable.
Principles for obtaining equivalent systems of equations.
(a): Changing the order in which the equations are listed produces an equivalent system.
(b): Suppose that in a system of equations, we multiply both sides of a single equation by a nonzero number (leaving the other equations) unchanged. Then the resulting system of equations is equivalent to the original system.
(c): Suppose that in a system of equations, we add a multiple of one equation to another equation (leaving the other equations) unchanged. Then the resulting system of equations is equivalent to the original system.
Method of factorization.
Suppose we wish to solve \(3x^2 = 27\). We begin by writing this in the standard form of a quadratic equation by subtracting 27 from each side to give \(3x^2 − 27 = 0\).
We now look for common factors. By observation, there is a common factor of 3 in both terms. This factor is extracted and written outside a pair of brackets. The contents of the brackets are adjusted accordingly:
\(3x^2 − 27 = 3(x^2 − 9) = 0\)
Notice here the difference between the two squares which can be factorized as
\(3(x^2 − 9) = 3(x − 3)(x + 3) = 0\)
If two quantities are multiplied together and the result is zero then either or both of the quantities must be zero. So either
\(x − 3 = 0\) or \(x + 3 = 0\)
so that
\(x = 3\) or \(x = −3
\)
These are the two solutions to the equation.
Method by completing the square.
Suppose we wish to solve \(x^2 − 3x − 2 = 0\).
In order to complete the square we look at the first two terms, and try to write them in the form \((?)^2\). Clearly, we need an x in the brackets:
\((x + ?)^2\) because when the term in brackets is squared this will give the term \(x^2\)
We also need the number \(− \frac{3}{2}\), which is half of the coefficient of x in the quadratic equation, \((x-\frac{3}{2})^2\) because when the term in brackets is squared this will give the term \(− 3x\)
However, removing the brackets from \((x-\frac{3}{2})^2\) we see there is also a term \((-\frac{3}{2})^2\) which we do not want, and so we subtract this again. So the quadratic equation can be written
\(x^2 − 3x − 2 = (x-\frac{3}{2})^2 - (-\frac{3}{2})^2 -2 = 0\)
after simplifying
We can write these solutions as
\(x=\frac{3+\sqrt{17}}{2}\) or \(\frac{3-\sqrt{17}}{2}\)
Again we have two answers. These are exact answers. Approximate values can be obtained using a calculator.
Method Solving quadratic equations by using graphs
In this section we will see how graphs can be used to solve quadratic equations. Whether the coefficient of \(x^2\) in the quadratic expression \(ax^2 + bx + c\) is positive then a graph of \(y = ax^2 + bx + c\) will take the form left. If the coefficient of \(x^2\) is negative the graph will take the form on the right.
What can happen when we plot a graph of \(y = ax^2 + bx + c\) for the case in which \(a\) is positive.
The horizontal line, the x axis, corresponds to points on the graph where y = 0. So points where the graph touches or crosses this axis correspond to solutions of \(ax^2 + bx + c = 0\).
The graph in (a) never cuts or touches the horizontal axis and so this corresponds to a quadratic equation \(ax^2 + bx + c = 0\) having no real roots.
The graph in (b) just touches the horizontal axis corresponding to the case in which the quadratic equation has two equal roots, also called ‘repeated roots’.
The graph in (c) cuts the horizontal axis twice, corresponding to the case in which the quadratic equation has two different roots.
What can happen when we plot a graph of \(y = ax^2 + bx + c\) for the case in which \(a\) is negative.
In case (a) there are no real roots. In case (b) there will be repeated roots. Case (c) corresponds to there being two real roots.
Method using the Quadratic formula.
Consider the general quadratic equation \(ax^2 + bx + c = 0\).
There is a formula for solving this: \(x =\frac{−b ± \sqrt{b^2-4ac}}{2a}\)
Nature of Roots of Quadratic Equation
The following resources were consulted in the design of this handout:
Show: ::
Attachment:
slide_1.jpg [ 89.12 KiB | Viewed 13083 times ]
Attachment:
GRE Equations.jpg [ 14.64 KiB | Viewed 47605 times ]
Attachment:
nature of roots.jpg [ 59.96 KiB | Viewed 16674 times ]
Attachment:
GRE equations on the graph.jpg [ 11.59 KiB | Viewed 12491 times ]
Attachment:
GRe equations axis.jpg [ 38.58 KiB | Viewed 12635 times ]
Attachment:
GRE equations graph.jpg [ 38.06 KiB | Viewed 12609 times ]
GRE Math Essentials - Equations
[#permalink]
 10 Apr 2025, 01:17
10 Apr 2025, 01:17
Expert Reply
Other Algebraic Properties
1.
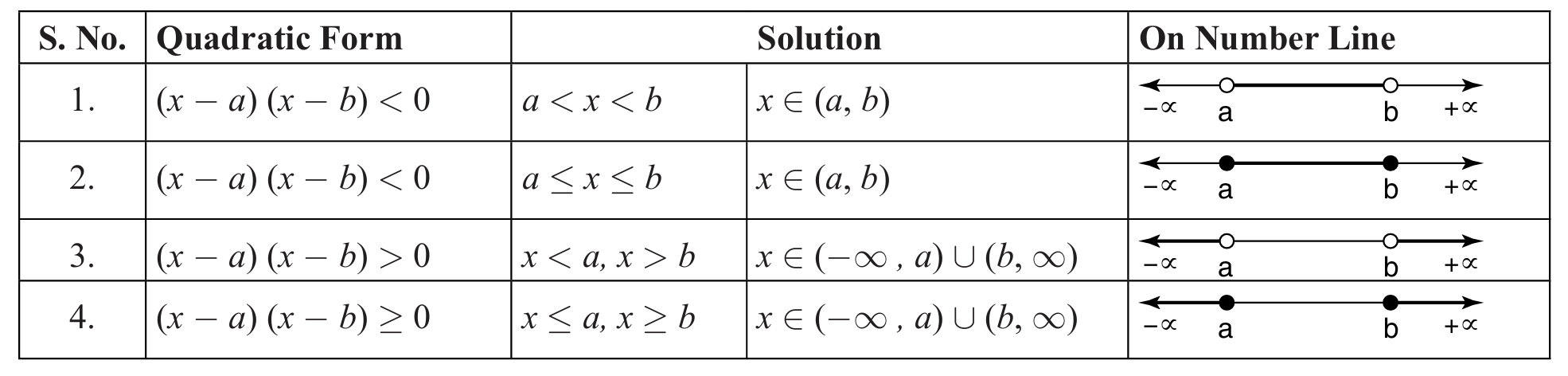
If $\(a<b\)$, the following relations holds true.

2.
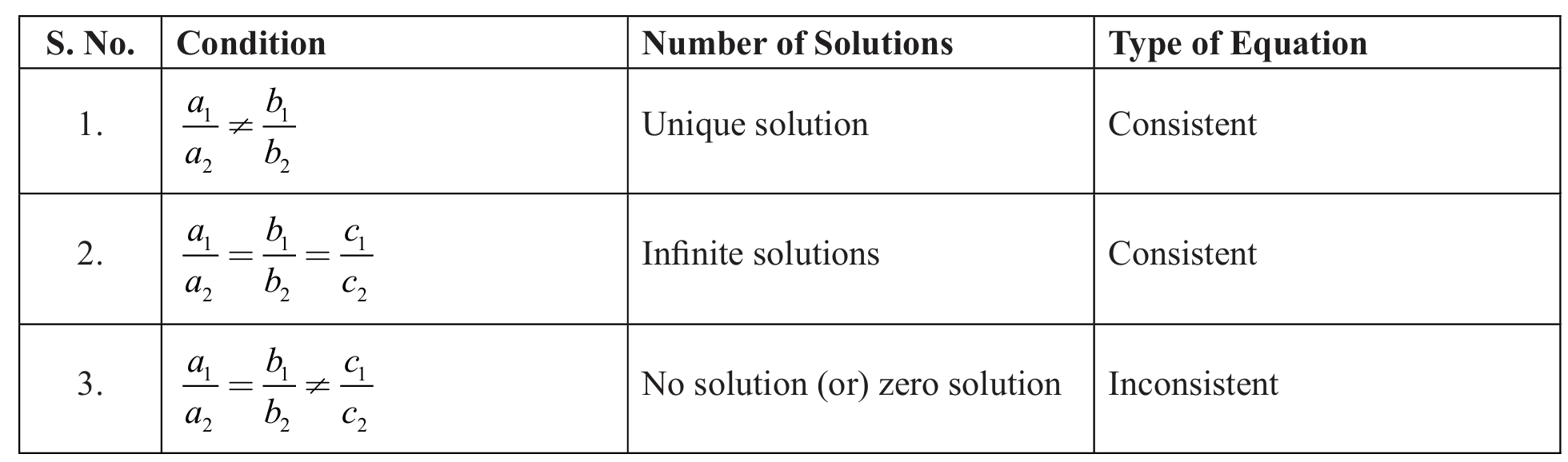
\(\text { If } a_1 x+b_1 y+c_1=0 \& a_2 x+b_2 y+c_2=0 \text {, the conditions for the number of solution is related as follows }\)

GRE algebra identities inequalities.png [ 78.63 KiB | Viewed 1938 times ]
GRE inequality properties.png [ 67.1 KiB | Viewed 1930 times ]
1.
If $\(a<b\)$, the following relations holds true.
2.
\(\text { If } a_1 x+b_1 y+c_1=0 \& a_2 x+b_2 y+c_2=0 \text {, the conditions for the number of solution is related as follows }\)
Show: ::
Attachment:
GRE algebra identities inequalities.png [ 78.63 KiB | Viewed 1938 times ]
Attachment:
GRE inequality properties.png [ 67.1 KiB | Viewed 1930 times ]

gmatclubot
GRE Math Essentials - Equations [#permalink]
10 Apr 2025, 01:17
Moderators:

