
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
GRE Quant - Integers Theory
[#permalink]
 05 Jun 2020, 01:10
05 Jun 2020, 01:10
13
Expert Reply
9
Bookmarks
Frequency of the concepts tested: Very High
GRE Number Types
GRE deals with only Real Numbers: Integers, Fractions and Irrational Numbers.
GRE deals with only Real Numbers: Integers, Fractions and Irrational Numbers.
INTEGERS
Definition
Integers are defined as: all negative natural numbers \(\{...,-4, -3, -2, -1\}\), zero \(\{0\}\), and positive natural numbers \(\{1, 2, 3, 4, ...\}\).
Note that integers do not include decimals or fractions - just whole numbers.
Even and Odd Numbers
An even number is an integer that is "evenly divisible" by 2, i.e., divisible by 2 without a remainder.
An even number is an integer of the form \(n=2k\), where \(k\) is an integer.
An odd number is an integer that is not evenly divisible by 2.
An odd number is an integer of the form \(n=2k+1\), where \(k\) is an integer.
Zero is an even number.
Addition / Subtraction:
even +/- even = even;
even +/- odd = odd;
odd +/- odd = even.
Multiplication:
even * even = even;
even * odd = even;
odd * odd = odd.
Division of two integers can result into an even/odd integer or a fraction.
ZERO:
1. 0 is an integer.
2. 0 is an even integer. An even number is an integer that is "evenly divisible" by 2, i.e., divisible by 2 without a remainder and as zero is evenly divisible by 2 then it must be even.
3. 0 is neither positive nor negative integer (the only one of this kind).
4. 0 is divisible by EVERY integer except 0 itself.
Definition
Integers are defined as: all negative natural numbers \(\{...,-4, -3, -2, -1\}\), zero \(\{0\}\), and positive natural numbers \(\{1, 2, 3, 4, ...\}\).
Note that integers do not include decimals or fractions - just whole numbers.
Even and Odd Numbers
An even number is an integer that is "evenly divisible" by 2, i.e., divisible by 2 without a remainder.
An even number is an integer of the form \(n=2k\), where \(k\) is an integer.
An odd number is an integer that is not evenly divisible by 2.
An odd number is an integer of the form \(n=2k+1\), where \(k\) is an integer.
Zero is an even number.
Addition / Subtraction:
even +/- even = even;
even +/- odd = odd;
odd +/- odd = even.
Multiplication:
even * even = even;
even * odd = even;
odd * odd = odd.
Division of two integers can result into an even/odd integer or a fraction.
ZERO:
1. 0 is an integer.
2. 0 is an even integer. An even number is an integer that is "evenly divisible" by 2, i.e., divisible by 2 without a remainder and as zero is evenly divisible by 2 then it must be even.
3. 0 is neither positive nor negative integer (the only one of this kind).
4. 0 is divisible by EVERY integer except 0 itself.
IRRATIONAL NUMBERS
Fractions (also known as rational numbers) can be written as terminating (ending) or repeating decimals (such as 0.5, 0.76, or 0.333333....). On the other hand, all those numbers that can be written as non-terminating, non-repeating decimals are non-rational, so they are called the "irrationals". Examples would be \(\sqrt{2}\) ("the square root of two") or the number pi (\(\pi=\)~3.14159..., from geometry). The rationals and the irrationals are two totally separate number types: there is no overlap.
Putting these two major classifications, the rationals and the irrationals, together in one set gives you the "real" numbers.
Fractions (also known as rational numbers) can be written as terminating (ending) or repeating decimals (such as 0.5, 0.76, or 0.333333....). On the other hand, all those numbers that can be written as non-terminating, non-repeating decimals are non-rational, so they are called the "irrationals". Examples would be \(\sqrt{2}\) ("the square root of two") or the number pi (\(\pi=\)~3.14159..., from geometry). The rationals and the irrationals are two totally separate number types: there is no overlap.
Putting these two major classifications, the rationals and the irrationals, together in one set gives you the "real" numbers.
POSITIVE AND NEGATIVE NUMBERS

The numbers to the right of zero are called positive numbers and those to the left are called negative. The first ones must be written with the positive (+) sign in front and the second ones with the negative sign in front (-).These numbers are also identified as signed numbers.
Important facts are also being true and are worth remembering as cold.
1) for any number a, one and only one of the following statements are true
A positive number is a real number that is greater than zero.
A negative number is a real number that is smaller than zero.
Zero is not positive, nor negative.
Right away is important to denote that the absolute value of a number a, | a |, is the distance between a and zero on the number line, both on the positive side and negative one. The number a, for instance, 5, is 5 units apart from zero and -5 is 5 units apart from zero on the negative side. As such, their absolute value is |5|. In this scenario 5 and - 5 are opposite numbers. The only number that is equal to its opposite is zero
2) The product of any number * zero is zero. And if the product of two numbers is zero, then at least one of the two is zero.
We need to consider that our numbers on the number line, and we are talking not only of integers but also NOT integers, can be the product or quotient of two positive numbers or two negative ones; or a mix: one positive or one negative.
3) The product and quotient of two positive numbers or two negative numbers are positive; the product and quotiet of a positive number and a negative number are negative.
Multiplication:
positive * positive = positive
positive * negative = negative
negative * negative = positive
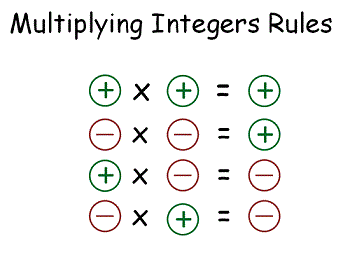
Division:
positive / positive = positive
positive / negative = negative
negative / negative = positive


The numbers to the right of zero are called positive numbers and those to the left are called negative. The first ones must be written with the positive (+) sign in front and the second ones with the negative sign in front (-).These numbers are also identified as signed numbers.
Important facts are also being true and are worth remembering as cold.
1) for any number a, one and only one of the following statements are true
- a is negative
- a is = to zero
- a is positive
A positive number is a real number that is greater than zero.
A negative number is a real number that is smaller than zero.
Zero is not positive, nor negative.
Right away is important to denote that the absolute value of a number a, | a |, is the distance between a and zero on the number line, both on the positive side and negative one. The number a, for instance, 5, is 5 units apart from zero and -5 is 5 units apart from zero on the negative side. As such, their absolute value is |5|. In this scenario 5 and - 5 are opposite numbers. The only number that is equal to its opposite is zero
2) The product of any number * zero is zero. And if the product of two numbers is zero, then at least one of the two is zero.
We need to consider that our numbers on the number line, and we are talking not only of integers but also NOT integers, can be the product or quotient of two positive numbers or two negative ones; or a mix: one positive or one negative.
3) The product and quotient of two positive numbers or two negative numbers are positive; the product and quotiet of a positive number and a negative number are negative.
Multiplication:
positive * positive = positive
positive * negative = negative
negative * negative = positive
Division:
positive / positive = positive
positive / negative = negative
negative / negative = positive
Prime Numbers
A Prime number is a natural number with exactly two distinct natural number divisors: 1 and itself. Otherwise a number is called a composite number. Therefore, 1 is not a prime, since it only has one divisor, namely 1. A number \(n > 1\) is prime if it cannot be written as a product of two factors \(a\) and \(b\), both of which are greater than 1: n = ab.
• The first twenty-six prime numbers are:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, 101
• Note: only positive numbers can be primes.
• There are infinitely many prime numbers.
• The only even prime number is 2, since any larger even number is divisible by 2. Also 2 is the smallest prime.
• All prime numbers except 2 and 5 end in 1, 3, 7 or 9, since numbers ending in 0, 2, 4, 6 or 8 are multiples of 2 and numbers ending in 0 or 5 are multiples of 5. Similarly, all prime numbers above 3 are of the form \(6n-1\) or \(6n+1\), because all other numbers are divisible by 2 or 3.
• Any nonzero natural number \(n\) can be factored into primes, written as a product of primes or powers of primes. Moreover, this factorization is unique except for a possible reordering of the factors.
• Prime factorization: every positive integer greater than 1 can be written as a product of one or more prime integers in a way which is unique. For instance integer \(n\) with three unique prime factors \(a\), \(b\), and \(c\) can be expressed as \(n=a^p*b^q*c^r\), where \(p\), \(q\), and \(r\) are powers of \(a\), \(b\), and \(c\), respectively and are \(\geq1\).
Example: \(4200=2^3*3*5^2*7\).
• Verifying the primality (checking whether the number is a prime) of a given number \(n\) can be done by trial division, that is to say dividing \(n\) by all integer numbers smaller than \(\sqrt{n}\), thereby checking whether \(n\) is a multiple of \(m\leq{\sqrt{n}}\).
Example: Verifying the primality of \(161\): \(\sqrt{161}\) is little less than \(13\), from integers from \(2\) to \(13\), \(161\) is divisible by \(7\), hence \(161\) is not prime.
Note that, it is only necessary to try dividing by prime numbers up to \(\sqrt{n}\), since if n has any divisors at all (besides 1 and n), then it must have a prime divisor.
• If \(n\) is a positive integer greater than 1, then there is always a prime number \(p\) with \(n < p < 2n\).
A Prime number is a natural number with exactly two distinct natural number divisors: 1 and itself. Otherwise a number is called a composite number. Therefore, 1 is not a prime, since it only has one divisor, namely 1. A number \(n > 1\) is prime if it cannot be written as a product of two factors \(a\) and \(b\), both of which are greater than 1: n = ab.
• The first twenty-six prime numbers are:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, 101
• Note: only positive numbers can be primes.
• There are infinitely many prime numbers.
• The only even prime number is 2, since any larger even number is divisible by 2. Also 2 is the smallest prime.
• All prime numbers except 2 and 5 end in 1, 3, 7 or 9, since numbers ending in 0, 2, 4, 6 or 8 are multiples of 2 and numbers ending in 0 or 5 are multiples of 5. Similarly, all prime numbers above 3 are of the form \(6n-1\) or \(6n+1\), because all other numbers are divisible by 2 or 3.
• Any nonzero natural number \(n\) can be factored into primes, written as a product of primes or powers of primes. Moreover, this factorization is unique except for a possible reordering of the factors.
• Prime factorization: every positive integer greater than 1 can be written as a product of one or more prime integers in a way which is unique. For instance integer \(n\) with three unique prime factors \(a\), \(b\), and \(c\) can be expressed as \(n=a^p*b^q*c^r\), where \(p\), \(q\), and \(r\) are powers of \(a\), \(b\), and \(c\), respectively and are \(\geq1\).
Example: \(4200=2^3*3*5^2*7\).
• Verifying the primality (checking whether the number is a prime) of a given number \(n\) can be done by trial division, that is to say dividing \(n\) by all integer numbers smaller than \(\sqrt{n}\), thereby checking whether \(n\) is a multiple of \(m\leq{\sqrt{n}}\).
Example: Verifying the primality of \(161\): \(\sqrt{161}\) is little less than \(13\), from integers from \(2\) to \(13\), \(161\) is divisible by \(7\), hence \(161\) is not prime.
Note that, it is only necessary to try dividing by prime numbers up to \(\sqrt{n}\), since if n has any divisors at all (besides 1 and n), then it must have a prime divisor.
• If \(n\) is a positive integer greater than 1, then there is always a prime number \(p\) with \(n < p < 2n\).
Factors
A divisor of an integer \(n\), also called a factor of \(n\), is an integer which evenly divides \(n\) without leaving a remainder. In general, it is said \(m\) is a factor of \(n\), for non-zero integers \(m\) and \(n\), if there exists an integer \(k\) such that \(n = km\).
• 1 (and -1) are divisors of every integer.
• Every integer is a divisor of itself.
• Every integer is a divisor of 0, except, by convention, 0 itself.
• Numbers divisible by 2 are called even and numbers not divisible by 2 are called odd.
• A positive divisor of n which is different from n is called a proper divisor.
• An integer n > 1 whose only proper divisor is 1 is called a prime number. Equivalently, one would say that a prime number is one which has exactly two factors: 1 and itself.
• Any positive divisor of n is a product of prime divisors of n raised to some power.
• If a number equals the sum of its proper divisors, it is said to be a perfect number.
Example: The proper divisors of 6 are 1, 2, and 3: 1+2+3=6, hence 6 is a perfect number.
There are some elementary rules:
• If \(a\) is a factor of \(b\) and \(a\) is a factor of \(c\), then \(a\) is a factor of \((b + c)\). In fact, \(a\) is a factor of \((mb + nc)\) for all integers \(m\) and \(n\).
• If \(a\) is a factor of \(b\) and \(b\) is a factor of \(c\), then \(a\) is a factor of \(c\).
• If \(a\) is a factor of \(b\) and \(b\) is a factor of \(a\), then \(a = b\) or \(a=-b\).
• If \(a\) is a factor of \(bc\), and \(gcd(a,b)=1\), then a is a factor of \(c\).
• If \(p\) is a prime number and \(p\) is a factor of \(ab\) then \(p\) is a factor of \(a\) or \(p\) is a factor of \(b\).
Finding the Number of Factors of an Integer
First make prime factorization of an integer \(n=a^p*b^q*c^r\), where \(a\), \(b\), and \(c\) are prime factors of \(n\) and \(p\), \(q\), and \(r\) are their powers.
The number of factors of \(n\) will be expressed by the formula \((p+1)(q+1)(r+1)\). NOTE: this will include 1 and n itself.
Example: Finding the number of all factors of 450: \(450=2^1*3^2*5^2\)
Total number of factors of 450 including 1 and 450 itself is \((1+1)*(2+1)*(2+1)=2*3*3=18\) factors.
Greatest Common Factor (Divisior) - GCF (GCD)
The greatest common divisor (gcd), also known as the greatest common factor (gcf), or highest common factor (hcf), of two or more non-zero integers, is the largest positive integer that divides the numbers without a remainder.
To find the GCF, you will need to do prime-factorization. Then, multiply the common factors (pick the lowest power of the common factors).
• Every common divisor of a and b is a divisor of gcd(a, b).
• a*b=gcd(a, b)*lcm(a, b)
Lowest Common Multiple - LCM
The lowest common multiple or lowest common multiple (lcm) or smallest common multiple of two integers a and b is the smallest positive integer that is a multiple both of a and of b. Since it is a multiple, it can be divided by a and b without a remainder. If either a or b is 0, so that there is no such positive integer, then lcm(a, b) is defined to be zero.
To find the LCM, you will need to do prime-factorization. Then multiply all the factors (pick the highest power of the common factors).
Perfect Square
A perfect square, is an integer that can be written as the square of some other integer. For example 16=4^2, is an perfect square.
There are some tips about the perfect square:
• The number of distinct factors of a perfect square is ALWAYS ODD.
• The sum of distinct factors of a perfect square is ALWAYS ODD.
• A perfect square ALWAYS has an ODD number of Odd-factors, and EVEN number of Even-factors.
• Perfect square always has even number of powers of prime factors.
Divisibility Rules Bonus Post - How to Solve: Divisibility Rules
2 - If the last digit is even, the number is divisible by 2.
3 - If the sum of the digits is divisible by 3, the number is also.
4 - If the last two digits form a number divisible by 4, the number is also.
5 - If the last digit is a 5 or a 0, the number is divisible by 5.
6 - If the number is divisible by both 3 and 2, it is also divisible by 6.
7 - Take the last digit, double it, and subtract it from the rest of the number, if the answer is divisible by 7 (including 0), then the number is divisible by 7.
8 - If the last three digits of a number are divisible by 8, then so is the whole number.
9 - If the sum of the digits is divisible by 9, so is the number.
10 - If the number ends in 0, it is divisible by 10.
11 - If you sum every second digit and then subtract all other digits and the answer is: 0, or is divisible by 11, then the number is divisible by 11.
Example: to see whether 9,488,699 is divisible by 11, sum every second digit: 4+8+9=21, then subtract the sum of other digits: 21-(9+8+6+9)=-11, -11 is divisible by 11, hence 9,488,699 is divisible by 11.
12 - If the number is divisible by both 3 and 4, it is also divisible by 12.
25 - Numbers ending with 00, 25, 50, or 75 represent numbers divisible by 25.
A divisor of an integer \(n\), also called a factor of \(n\), is an integer which evenly divides \(n\) without leaving a remainder. In general, it is said \(m\) is a factor of \(n\), for non-zero integers \(m\) and \(n\), if there exists an integer \(k\) such that \(n = km\).
• 1 (and -1) are divisors of every integer.
• Every integer is a divisor of itself.
• Every integer is a divisor of 0, except, by convention, 0 itself.
• Numbers divisible by 2 are called even and numbers not divisible by 2 are called odd.
• A positive divisor of n which is different from n is called a proper divisor.
• An integer n > 1 whose only proper divisor is 1 is called a prime number. Equivalently, one would say that a prime number is one which has exactly two factors: 1 and itself.
• Any positive divisor of n is a product of prime divisors of n raised to some power.
• If a number equals the sum of its proper divisors, it is said to be a perfect number.
Example: The proper divisors of 6 are 1, 2, and 3: 1+2+3=6, hence 6 is a perfect number.
There are some elementary rules:
• If \(a\) is a factor of \(b\) and \(a\) is a factor of \(c\), then \(a\) is a factor of \((b + c)\). In fact, \(a\) is a factor of \((mb + nc)\) for all integers \(m\) and \(n\).
• If \(a\) is a factor of \(b\) and \(b\) is a factor of \(c\), then \(a\) is a factor of \(c\).
• If \(a\) is a factor of \(b\) and \(b\) is a factor of \(a\), then \(a = b\) or \(a=-b\).
• If \(a\) is a factor of \(bc\), and \(gcd(a,b)=1\), then a is a factor of \(c\).
• If \(p\) is a prime number and \(p\) is a factor of \(ab\) then \(p\) is a factor of \(a\) or \(p\) is a factor of \(b\).
Finding the Number of Factors of an Integer
First make prime factorization of an integer \(n=a^p*b^q*c^r\), where \(a\), \(b\), and \(c\) are prime factors of \(n\) and \(p\), \(q\), and \(r\) are their powers.
The number of factors of \(n\) will be expressed by the formula \((p+1)(q+1)(r+1)\). NOTE: this will include 1 and n itself.
Example: Finding the number of all factors of 450: \(450=2^1*3^2*5^2\)
Total number of factors of 450 including 1 and 450 itself is \((1+1)*(2+1)*(2+1)=2*3*3=18\) factors.
Greatest Common Factor (Divisior) - GCF (GCD)
The greatest common divisor (gcd), also known as the greatest common factor (gcf), or highest common factor (hcf), of two or more non-zero integers, is the largest positive integer that divides the numbers without a remainder.
To find the GCF, you will need to do prime-factorization. Then, multiply the common factors (pick the lowest power of the common factors).
• Every common divisor of a and b is a divisor of gcd(a, b).
• a*b=gcd(a, b)*lcm(a, b)
Lowest Common Multiple - LCM
The lowest common multiple or lowest common multiple (lcm) or smallest common multiple of two integers a and b is the smallest positive integer that is a multiple both of a and of b. Since it is a multiple, it can be divided by a and b without a remainder. If either a or b is 0, so that there is no such positive integer, then lcm(a, b) is defined to be zero.
To find the LCM, you will need to do prime-factorization. Then multiply all the factors (pick the highest power of the common factors).
Perfect Square
A perfect square, is an integer that can be written as the square of some other integer. For example 16=4^2, is an perfect square.
There are some tips about the perfect square:
• The number of distinct factors of a perfect square is ALWAYS ODD.
• The sum of distinct factors of a perfect square is ALWAYS ODD.
• A perfect square ALWAYS has an ODD number of Odd-factors, and EVEN number of Even-factors.
• Perfect square always has even number of powers of prime factors.
Divisibility Rules Bonus Post - How to Solve: Divisibility Rules
2 - If the last digit is even, the number is divisible by 2.
3 - If the sum of the digits is divisible by 3, the number is also.
4 - If the last two digits form a number divisible by 4, the number is also.
5 - If the last digit is a 5 or a 0, the number is divisible by 5.
6 - If the number is divisible by both 3 and 2, it is also divisible by 6.
7 - Take the last digit, double it, and subtract it from the rest of the number, if the answer is divisible by 7 (including 0), then the number is divisible by 7.
8 - If the last three digits of a number are divisible by 8, then so is the whole number.
9 - If the sum of the digits is divisible by 9, so is the number.
10 - If the number ends in 0, it is divisible by 10.
11 - If you sum every second digit and then subtract all other digits and the answer is: 0, or is divisible by 11, then the number is divisible by 11.
Example: to see whether 9,488,699 is divisible by 11, sum every second digit: 4+8+9=21, then subtract the sum of other digits: 21-(9+8+6+9)=-11, -11 is divisible by 11, hence 9,488,699 is divisible by 11.
12 - If the number is divisible by both 3 and 4, it is also divisible by 12.
25 - Numbers ending with 00, 25, 50, or 75 represent numbers divisible by 25.
Consecutive Integers
Consecutive integers are integers that follow one another, without skipping any integers. 7, 8, 9, and -2, -1, 0, 1, are consecutive integers.
• Sum of \(n\) consecutive integers equals the mean multiplied by the number of terms, \(n\). Given consecutive integers \(\{-3, -2, -1, 0, 1,2\}\), \(mean=\frac{-3+2}{2}=-\frac{1}{2}\), (mean equals to the average of the first and last terms), so the sum equals to \(-\frac{1}{2}*6=-3\).
• If n is odd, the sum of consecutive integers is always divisible by n. Given \(\{9,10,11\}\), we have \(n=3\) consecutive integers. The sum of 9+10+11=30, therefore, is divisible by 3.
• If n is even, the sum of consecutive integers is never divisible by n. Given \(\{9,10,11,12\}\), we have \(n=4\) consecutive integers. The sum of 9+10+11+12=42, therefore, is not divisible by 4.
• The product of \(n\) consecutive integers is always divisible by \(n!\).
Given \(n=4\) consecutive integers: \(\{3,4,5,6\}\). The product of 3*4*5*6 is 360, which is divisible by 4!=24.
Evenly Spaced Set
Evenly spaced set or an arithmetic progression is a sequence of numbers such that the difference of any two successive members of the sequence is a constant. The set of integers \(\{9,13,17,21\}\) is an example of evenly spaced set. Set of consecutive integers is also an example of evenly spaced set.
• If the first term is \(a_1\) and the common difference of successive members is \(d\), then the \(n_{th}\) term of the sequence is given by:
\(a_ n=a_1+d(n-1)\)
• In any evenly spaced set the arithmetic mean (average) is equal to the median and can be calculated by the formula \(mean=median=\frac{a_1+a_n}{2}\), where \(a_1\) is the first term and \(a_n\) is the last term. Given the set \(\{7,11,15,19\}\), \(mean=median=\frac{7+19}{2}=13\).
• The sum of the elements in any evenly spaced set is given by:
\(Sum=\frac{a_1+a_n}{2}*n\), the mean multiplied by the number of terms. OR, \(Sum=\frac{2a_1+d(n-1)}{2}*n\)
• Special cases:
Sum of n first positive integers: \(1+2+...+n=\frac{1+n}{2}*n\)
Sum of n first positive odd numbers: \(a_1+a_2+...+a_n=1+3+...+a_n=n^2\), where \(a_n\) is the last, \(n_{th}\) term and given by: \(a_n=2n-1\). Given \(n=5\) first odd positive integers, then their sum equals to \(1+3+5+7+9=5^2=25\).
Sum of n first positive even numbers: \(a_1+a_2+...+a_n=2+4+...+a_n\)\(=n(n+1)\), where \(a_n\) is the last, \(n_{th}\) term and given by: \(a_n=2n\). Given \(n=4\) first positive even integers, then their sum equals to \(2+4+6+8=4(4+1)=20\).
• If the evenly spaced set contains odd number of elements, the mean is the middle term, so the sum is middle term multiplied by number of terms. There are five terms in the set {1, 7, 13, 19, 25}, middle term is 13, so the sum is 13*5 =65.
Consecutive integers are integers that follow one another, without skipping any integers. 7, 8, 9, and -2, -1, 0, 1, are consecutive integers.
• Sum of \(n\) consecutive integers equals the mean multiplied by the number of terms, \(n\). Given consecutive integers \(\{-3, -2, -1, 0, 1,2\}\), \(mean=\frac{-3+2}{2}=-\frac{1}{2}\), (mean equals to the average of the first and last terms), so the sum equals to \(-\frac{1}{2}*6=-3\).
• If n is odd, the sum of consecutive integers is always divisible by n. Given \(\{9,10,11\}\), we have \(n=3\) consecutive integers. The sum of 9+10+11=30, therefore, is divisible by 3.
• If n is even, the sum of consecutive integers is never divisible by n. Given \(\{9,10,11,12\}\), we have \(n=4\) consecutive integers. The sum of 9+10+11+12=42, therefore, is not divisible by 4.
• The product of \(n\) consecutive integers is always divisible by \(n!\).
Given \(n=4\) consecutive integers: \(\{3,4,5,6\}\). The product of 3*4*5*6 is 360, which is divisible by 4!=24.
Evenly Spaced Set
Evenly spaced set or an arithmetic progression is a sequence of numbers such that the difference of any two successive members of the sequence is a constant. The set of integers \(\{9,13,17,21\}\) is an example of evenly spaced set. Set of consecutive integers is also an example of evenly spaced set.
• If the first term is \(a_1\) and the common difference of successive members is \(d\), then the \(n_{th}\) term of the sequence is given by:
\(a_ n=a_1+d(n-1)\)
• In any evenly spaced set the arithmetic mean (average) is equal to the median and can be calculated by the formula \(mean=median=\frac{a_1+a_n}{2}\), where \(a_1\) is the first term and \(a_n\) is the last term. Given the set \(\{7,11,15,19\}\), \(mean=median=\frac{7+19}{2}=13\).
• The sum of the elements in any evenly spaced set is given by:
\(Sum=\frac{a_1+a_n}{2}*n\), the mean multiplied by the number of terms. OR, \(Sum=\frac{2a_1+d(n-1)}{2}*n\)
• Special cases:
Sum of n first positive integers: \(1+2+...+n=\frac{1+n}{2}*n\)
Sum of n first positive odd numbers: \(a_1+a_2+...+a_n=1+3+...+a_n=n^2\), where \(a_n\) is the last, \(n_{th}\) term and given by: \(a_n=2n-1\). Given \(n=5\) first odd positive integers, then their sum equals to \(1+3+5+7+9=5^2=25\).
Sum of n first positive even numbers: \(a_1+a_2+...+a_n=2+4+...+a_n\)\(=n(n+1)\), where \(a_n\) is the last, \(n_{th}\) term and given by: \(a_n=2n\). Given \(n=4\) first positive even integers, then their sum equals to \(2+4+6+8=4(4+1)=20\).
• If the evenly spaced set contains odd number of elements, the mean is the middle term, so the sum is middle term multiplied by number of terms. There are five terms in the set {1, 7, 13, 19, 25}, middle term is 13, so the sum is 13*5 =65.
ORDER OF OPERATIONS - PEMDAS
Perform the operations inside a Parenthesis first (absolute value signs also fall into this category), then Exponents, then Multiplication and Division, from left to right, then Addition and Subtraction, from left to right - PEMDAS.
Special cases:
• An exclamation mark indicates that one should compute the factorial of the term immediately to its left, before computing any of the lower-precedence operations, unless grouping symbols dictate otherwise. But \(3^2!\) means \((3^2)! = 9!\) while \(2^{5!} = 2^{120}\); a factorial in an exponent applies to the exponent, while a factorial not in the exponent applies to the entire power.
• If exponentiation is indicated by stacked symbols, the rule is to work from the top down, thus:
\(a^{m^n}=a^{(m^n)}\) and not \((a^m)^n\)
Perform the operations inside a Parenthesis first (absolute value signs also fall into this category), then Exponents, then Multiplication and Division, from left to right, then Addition and Subtraction, from left to right - PEMDAS.
Special cases:
• An exclamation mark indicates that one should compute the factorial of the term immediately to its left, before computing any of the lower-precedence operations, unless grouping symbols dictate otherwise. But \(3^2!\) means \((3^2)! = 9!\) while \(2^{5!} = 2^{120}\); a factorial in an exponent applies to the exponent, while a factorial not in the exponent applies to the entire power.
• If exponentiation is indicated by stacked symbols, the rule is to work from the top down, thus:
\(a^{m^n}=a^{(m^n)}\) and not \((a^m)^n\)
Show: ::
Attachment:
multiplying integers rules.gif [ 20.4 KiB | Viewed 72669 times ]
Attachment:
#greprepclub dividing integers rules.jpg [ 30.3 KiB | Viewed 71501 times ]
Re: GRE Quant - Integers Theory
[#permalink]
 11 Jun 2020, 05:23
11 Jun 2020, 05:23
2
Expert Reply
4
Bookmarks
Integers Questions by Tag
Quantitative Comparison Questions
Multiple-choice Questions — Select One Answer Choice
Multiple-choice Questions — Select One or More Answer Choices
Numeric Entry Question
Data Interpretation Sets
Moderator

Joined: 02 Jan 2020
Status:GRE Quant Tutor
Posts: 1141
Given Kudos: 9
Location: India
Concentration: General Management
Schools: XLRI Jamshedpur, India - Class of 2014
GMAT 1: 700 Q51 V31
GPA: 2.8
WE:Engineering (Computer Software)
Re: GRE Quant - Integers Theory
[#permalink]
 05 Jun 2020, 01:19
05 Jun 2020, 01:19
5
1
2
Bookmarks
How to Solve: LCM, GCD and Properties of LCM and GCD
What is LCM and how to find LCM of 2 or more numbers using three methods.
What is GCD and how to find GCD of 2 or more numbers using three methods.
• Listing Out Multiples
• Common Division Method
• Prime Factorization Method
How to find LCM and GCD of Exponents and Fractions.
Properties of LCM and GCD
Prop 1:
LCM of 0 and any number does not exist
• LCM ( 0 , a ) = Does not exist
Prop 2:
GCD of 0 and any number is equal to the number itself. (Because all whole numbers are factors of 0. So, a will be also be a factor of 0.)
• GCD ( 0 , a ) = a
Prop 3:
LCM of 1 and any number is equal to the number itself
• LCM ( 1 , a ) = a
Prop 4:
GCD of 1 and any number is equal to 1
• GCD ( 1 , a ) = 1
Prop 5:
Product of two numbers = Product of their LCM and GCD
• a*b = LCM(a,b) * GCD(a,b)
Prop 6:
LCM of two numbers is always a multiple of their GCD
• LCM(a,b) = GCD(a,b) * k [where k is an integer]
Prop 7:
LCM of two numbers always lies between the larger of the two numbers and the product of those two numbers
• Larger (a,b) <= LCM (a,b) <= a*b
Prop 8:
GCD of two numbers is always smaller than or equal to the smaller of those two numbers
• GCD (a,b) <= Smaller (a,b)
Prop 9:
LCM of two numbers is equal to the larger of the two numbers when one number is multiple of other
• LCM(a,b) = Larger(a,b) => either a is a multiple of b or b is a multiple of a
Prop 10:
LCM of two numbers is equal to the product of those two numbers when the numbers are co-prime
• LCM(a,b) = a*b => a and b are co-prime numbers
Co-prime numbers are numbers which have only one factor in common (i.e. 1)
Prop 11:
GCD of two numbers is equal to the smaller of the two numbers when one number is multiple of other
• GCD(a,b) = Smaller(a,b) => either a is a multiple of b or b is a multiple of a
Prop 12:
GCD of two numbers is 1 when the numbers are co-prime
• GCD(a,b)=1 => a and b are co-prime numbers
Prop 13:
All numbers can be written as a multiple of their GCD
• a and b can be written as a multiple of their GCD
• a = GCD(a,b) * k [where k is an integer]
• b = GCD(a,b) * t [where t is an integer]
Prop 14:
All multiples of LCM are divisible by the numbers whose LCM we have calculated
• Multiples of LCM(a,b) will be divisible by both a and b
Prop 15:
a + b and a -b will always be divisible by GCD(a,b)
• \(\frac{a+b}{GCD(a,b) }=k \) [where k is an integer]
• \(\frac{a-b}{GCD(a,b) }=t \) [where t is an integer]
Sample Word Problems:
Prob 1: Joe has 12 A-grade projects and Chris has 18 B-grade projects. They have to divide up the projects into small teams with equal number of projects in each team; each team can have either A-grade project or B-grade project only. If there is no remainder, find the largest possible number of projects in each team.
Prob 2: Joe and Chris were each given a piece of wood of equal length. Joe cuts his wood piece into equal lengths of 12 cm, while Chris cuts his wood piece into equal lengths of 18 cm. If there was no remainder in both cases, find the shortest possible length of wood given to them.
Prob 3: Two steel pieces of length 340 cm and 408 cm are to be cut into pieces of all the same length without remainder. Find the greatest possible length of the pieces.
Solutions:
Prob 1: GCD (12,18) =6
Prob 2: LCM(12,18) = 36
Prob 3: GCD(340,408) = 68
Hope it helps!


