
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
The figure above shows the graph of the function defined by
[#permalink]
 Updated on: 26 Jan 2019, 17:45
Updated on: 26 Jan 2019, 17:45
Please can anyone help with this question, the correct choice is E, how can we solve such questions.
Please look at the attached image.
thnxxxx

#GREpracticequestion The figure above shows the graph of the ftmctionf defined by.jpg [ 12.85 KiB | Viewed 1437 times ]
The figure above shows the graph of the function defined by \(f(x) = |2x| +4\) for all numbers x. For which of the following function g defined for all numbers x does the graph of g intersect the graph of \(f\) ?
A. \(g(x)=x — 2\)
B. \(g(x)=x + 3\)
C. \(g(x)= 2x — 2\)
D. \(g(x) = 2x + 3\)
E. \(g(x) = 3x — 2\)
Please look at the attached image.
thnxxxx
Attachment:
#GREpracticequestion The figure above shows the graph of the ftmctionf defined by.jpg [ 12.85 KiB | Viewed 1437 times ]
The figure above shows the graph of the function defined by \(f(x) = |2x| +4\) for all numbers x. For which of the following function g defined for all numbers x does the graph of g intersect the graph of \(f\) ?
A. \(g(x)=x — 2\)
B. \(g(x)=x + 3\)
C. \(g(x)= 2x — 2\)
D. \(g(x) = 2x + 3\)
E. \(g(x) = 3x — 2\)
Re: The figure above shows the graph of the function defined by
[#permalink]
 26 Jan 2019, 18:00
26 Jan 2019, 18:00
1
This is one of the sample question by ETS available in their website.
Here the main point to remember is sometimes you need to draw the figure to find the right answer.
First of all notice that the given eqn of the line has a slope of 2, option C and D also have a slope of 2 so we can put a value for x and see if any of the two lines will intersect the given eqn for \(f(x)\).
Let us take \(x = 1\) we get, for c: \(g(x) = 0\); for D: \(g(x) = 5\)\(\)
Since C and D do not intersect \(f(x)\) for \(x = 1\) we can conclude they will not intersect f(x) because f(x) and g(x) have the same slope.
option \(A\)and \(B\) are linear graph.
Let us draw the figure for option A in a graph
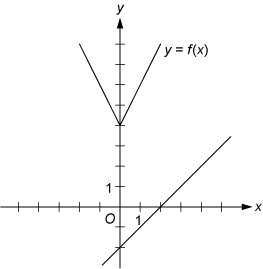
intersection.gif [ 2.27 KiB | Viewed 1465 times ]
This graph will not intersect line f(x) this is because slope of line f(x) is 2 whereas the slope of line g(x) is 1. For every increase in value of x f(x) will be more steeper than g(x). Choice B can be eliminated as well. This leaves us with choice E only.
Here the main point to remember is sometimes you need to draw the figure to find the right answer.
First of all notice that the given eqn of the line has a slope of 2, option C and D also have a slope of 2 so we can put a value for x and see if any of the two lines will intersect the given eqn for \(f(x)\).
Let us take \(x = 1\) we get, for c: \(g(x) = 0\); for D: \(g(x) = 5\)\(\)
Since C and D do not intersect \(f(x)\) for \(x = 1\) we can conclude they will not intersect f(x) because f(x) and g(x) have the same slope.
option \(A\)and \(B\) are linear graph.
Let us draw the figure for option A in a graph
Attachment:
intersection.gif [ 2.27 KiB | Viewed 1465 times ]
This graph will not intersect line f(x) this is because slope of line f(x) is 2 whereas the slope of line g(x) is 1. For every increase in value of x f(x) will be more steeper than g(x). Choice B can be eliminated as well. This leaves us with choice E only.
Re: The figure above shows the graph of the function defined by
[#permalink]
 27 Jan 2019, 11:51
27 Jan 2019, 11:51
Expert Reply
The question is discussed here
https://gre.myprepclub.com/forum/the-gure- ... -6322.html
Do a search on the board before to post a question. Especially if they are official question. We discussed all of them here on the board
Regards
https://gre.myprepclub.com/forum/the-gure- ... -6322.html
Do a search on the board before to post a question. Especially if they are official question. We discussed all of them here on the board
Regards






