GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Which of the following could be the third quartile value for number of
[#permalink]
27 Nov 2024, 01:21
27 Nov 2024, 01:21
Expert Reply
00:00
Question Stats:
60% (02:05) correct
40% (01:33) wrong based on 5 sessions
Hide Show timer Statistics
In a survey, 82 high school students were randomly selected and asked how many hours of television they had watched in the previous week. The histogram below displays their answers.
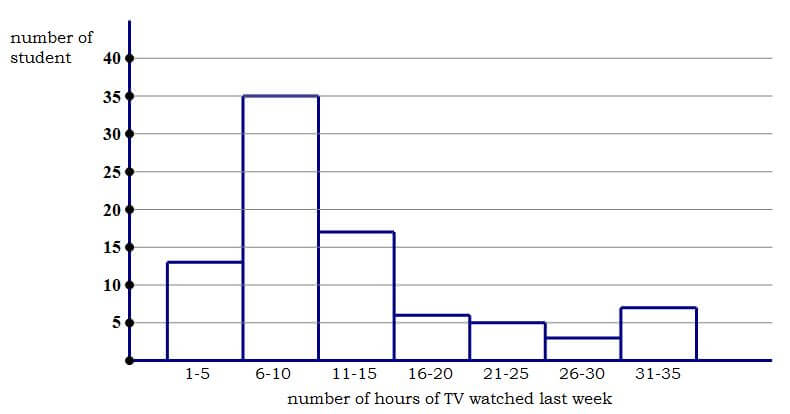
Which of the following could be the third quartile value for number of hours of TV watched last week?
A. 11
B. 17
C. 21
D. 23
E. 26
Which of the following could be the third quartile value for number of hours of TV watched last week?
A. 11
B. 17
C. 21
D. 23
E. 26
- Part of the project: GRE Quant & Verbal ADVANCED Daily Challenge 2024 Edition - Gain 20 Kudos & Get FREE Access to GRE Prep Club TESTS
- Also replying to the unanswered questions
Which of the following could be the third quartile value for number of
[#permalink]
21 Dec 2024, 13:22
21 Dec 2024, 13:22
Expert Reply
The third quartile is the 75th percentile, dividing the lower 3/4 from the upper 1/4. If we think of the median as dividing the whole list into an upper half and lower half, the third quartile is the median of that upper list.
This whole list has 82 numbers. The median would be the average of the 41st and 42nd numbers. The "upper list" would consist of 41 numbers, starting at the 42nd number and ending at the 82 number.
The number 41 can be expressed as 41 = 20 + 1 + 20--the median of the upper list, the third quartile of the whole list, will have 20 number of the upper list above it and 20 below it.
On that upper list, the first 20 numbers go from the 42nd number to the 61st number; the 62nd would be the median; and the last 20 numbers would go from the 63rd number to the 82nd number.
Where is the 62nd number on this list? Well, we could count up 62 places from the left side, or we could just count down 20 from the right side.
The rightmost column has 3 numbers. The two rightmost columns have 6 numbers. The three rightmost columns have 11 numbers.
The four rightmost columns have 17 numbers--still not quite enough. The five rightmost columns have 28 numbers-- these columns contain the third quartile.
It must be in the 11-15 column. The only number on the list in that column is 11, so Answer = (A).
This whole list has 82 numbers. The median would be the average of the 41st and 42nd numbers. The "upper list" would consist of 41 numbers, starting at the 42nd number and ending at the 82 number.
The number 41 can be expressed as 41 = 20 + 1 + 20--the median of the upper list, the third quartile of the whole list, will have 20 number of the upper list above it and 20 below it.
On that upper list, the first 20 numbers go from the 42nd number to the 61st number; the 62nd would be the median; and the last 20 numbers would go from the 63rd number to the 82nd number.
Where is the 62nd number on this list? Well, we could count up 62 places from the left side, or we could just count down 20 from the right side.
The rightmost column has 3 numbers. The two rightmost columns have 6 numbers. The three rightmost columns have 11 numbers.
The four rightmost columns have 17 numbers--still not quite enough. The five rightmost columns have 28 numbers-- these columns contain the third quartile.
It must be in the 11-15 column. The only number on the list in that column is 11, so Answer = (A).
Moderators:
