GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
A right angled triangle has base 1 unit and height of triangle is equa
[#permalink]
10 May 2023, 04:59
10 May 2023, 04:59
Expert Reply
1
Bookmarks
00:00
Question Stats:
90% (01:22) correct
9% (01:22) wrong based on 11 sessions
Hide Show timer Statistics
A right angled triangle has base 1 unit and height of triangle is equal to the diagnonal of the square of side 2 units. What is the value of perimeter, to the closest integer, of the triangle?
Post A Detailed Correct Solution For The Above Questions And Get A Kudos.
Question From Our Project: Free GRE Prep Club Tests in Exchange for 20 Kudos
\(\Longrightarrow\) GRE - Quant Daily Topic-wise Challenge
\(\Longrightarrow\) GRE GEOMETRY
Show: ::
7
Post A Detailed Correct Solution For The Above Questions And Get A Kudos.
Question From Our Project: Free GRE Prep Club Tests in Exchange for 20 Kudos
\(\Longrightarrow\) GRE - Quant Daily Topic-wise Challenge
\(\Longrightarrow\) GRE GEOMETRY
Re: A right angled triangle has base 1 unit and height of triangle is equa
[#permalink]
27 May 2023, 23:25
27 May 2023, 23:25
Expert Reply
OE
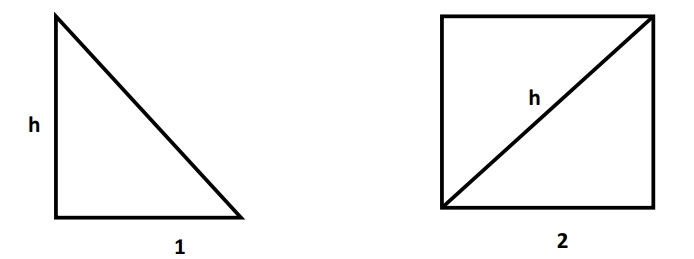
GRE triangles (4).jpg [ 21.79 KiB | Viewed 1321 times ]
We are given that the height of the triangle ABC = Diagonal of the square with side 2
units.
From the square, PR = h = 2√2 units (using Pythagoras theorem)
So, In triangle ABC, h = 2√2 units
By using Pythagoras theorem,
AC^2 = AB^2 + BC^2= (2√2))^2+ 1^2
AC^2 = 8 + 1 = 9
AC = 3 units.
So, the perimeter of the triangle ABC = 3 + 1 + 2√2 = 4 + 2√2 = 6.82 = 7 units (rounded off to nearest integer)
Ans. (7)
Attachment:
GRE triangles (4).jpg [ 21.79 KiB | Viewed 1321 times ]
We are given that the height of the triangle ABC = Diagonal of the square with side 2
units.
From the square, PR = h = 2√2 units (using Pythagoras theorem)
So, In triangle ABC, h = 2√2 units
By using Pythagoras theorem,
AC^2 = AB^2 + BC^2= (2√2))^2+ 1^2
AC^2 = 8 + 1 = 9
AC = 3 units.
So, the perimeter of the triangle ABC = 3 + 1 + 2√2 = 4 + 2√2 = 6.82 = 7 units (rounded off to nearest integer)
Ans. (7)
Re: A right angled triangle has base 1 unit and height of triangle is equa
[#permalink]
26 Oct 2024, 18:17
26 Oct 2024, 18:17
Hello from the GRE Prep Club BumpBot!
Thanks to another GRE Prep Club member, I have just discovered this valuable topic, yet it had no discussion for over a year. I am now bumping it up - doing my job. I think you may find it valuable (esp those replies with Kudos).
Want to see all other topics I dig out? Follow me (click follow button on profile). You will receive a summary of all topics I bump in your profile area as well as via email.
Thanks to another GRE Prep Club member, I have just discovered this valuable topic, yet it had no discussion for over a year. I am now bumping it up - doing my job. I think you may find it valuable (esp those replies with Kudos).
Want to see all other topics I dig out? Follow me (click follow button on profile). You will receive a summary of all topics I bump in your profile area as well as via email.
