
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
Coordinate Geometry Practice Question
[#permalink]
 09 Oct 2015, 12:13
09 Oct 2015, 12:13
5
Expert Reply
Question Stats:
 25% (00:24) correct
25% (00:24) correct
 75% (01:28) wrong
75% (01:28) wrong  based on 16 sessions
based on 16 sessions
Hide Show timer Statistics
In the xy-plane, line k is a line that does not pass through the origin.
Which of the following statements individually provide(s) sufficient additional information to determine whether the slope of line k is positive?
Indicate all such statements.
Which of the following statements individually provide(s) sufficient additional information to determine whether the slope of line k is positive?
Indicate all such statements.
- [A] The x-intercept of line k is the negative reciprocal of the y-intercept of line k.
[B] The product of the x-intercept and the y-intercept of line k is negative.
[C] Line k passes through the points (a, b) and (r, s), where (a − r)(b − s) > 0.
Show: :: OA
A, B, C
Re: Coordinate Geometry Practice Question
[#permalink]
 13 Oct 2015, 07:24
13 Oct 2015, 07:24
3
Expert Reply
RBornPowerScore wrote:
In the xy-plane, line k is a line that does not pass through the origin.
Which of the following statements individually provide(s) sufficient additional information to determine whether the slope of line k is positive?
Indicate all such statements.
Which of the following statements individually provide(s) sufficient additional information to determine whether the slope of line k is positive?
Indicate all such statements.
- [A] The x-intercept of line k is the negative reciprocal of the y-intercept of line k. Th
[B] The product of the x-intercept and the y-intercept of line k is negative.
[C] Line k passes through the points (a, b) and (r, s), where (a − r)(b − s) > 0.
Show: :: OA
A, B, C
Solution
Show: :: Answer
The best way to go about this is by using line equation \(\frac{x}{a} + \frac{y}{b} = 1\). Here a is x intercept and b is the y intercept.
- [A] Now take a = 1 so b = -1 so the line becomes \(\frac{x}{1} + \frac{y}{-1} = 1\). This line has a positive slope.
[B] Now take a = 1 so b = -1 so the line becomes \(\frac{x}{1} + \frac{y}{-1} = 1\). This line has a positive slope.
[C] This one is the toughest on the list. Given (a − r)(b − s) > 0; so (a − r)> 0 and (b − s)> 0 or (a − r)< 0 and (b − s)< 0. Now Slope of a line say (m) passing through points (a,b) and (r,s) is \(m = \frac{(b-s)}{(a-r)}\). Hence if the inequality holds good then the slope is always positive.
Re: Coordinate Geometry Practice Question
[#permalink]
 13 Oct 2015, 07:49
13 Oct 2015, 07:49
Expert Reply
Thanks to sandy for posting a solution. Here's another explanation of how to solve the problem.
Show: :: Solution
For each answer choice, ask yourself "Does this statement guarantee that line k has a positive slope?" If your reply is "Yes, the slope of line k must be positive if this statement is true" then select that answer choice.
Consider A and B. Drawing a figure like the one attached will help you evaluate these two statements. 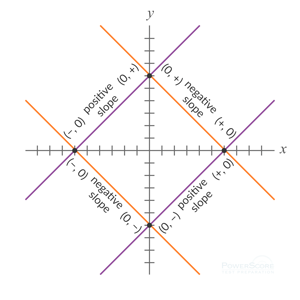
GRE-Math-Slope-Intercepts.png [ 20.82 KiB | Viewed 11238 times ]
For lines with positive slope that avoid the origin, the x- and y-intercepts must have opposite signs. For lines with negative slope that avoid the origin, the intercepts must have matching signs. If one intercept is the negative reciprocal of the other, then one intercept must be negative and the other positive. The same is true if the product of the intercepts is negative. So if A or B is true, then the slope of line k must be positive.
Now consider C. This statement gives two points on line k and says that if you multiply the difference in their y-coordinates by the difference in their x-coordinates, you get a positive value. But wait. If you divide those differences, you get the slope of line k. So Plug (a, b) and (r, s) into the slope formula: slope = (b − s) ÷ (a − r)
If (a − r)(b − s) > 0, then either both differences are positive or both are negative. And if (a − r) and (b − s) yield differences with matching signs, then (b − s) ÷ (a − r) > 0, meaning the slope of line k must be positive. So if C is true, then the slope of line k must be positive.
A, B, and C are all correct.
Consider A and B. Drawing a figure like the one attached will help you evaluate these two statements.
Attachment:
GRE-Math-Slope-Intercepts.png [ 20.82 KiB | Viewed 11238 times ]
Now consider C. This statement gives two points on line k and says that if you multiply the difference in their y-coordinates by the difference in their x-coordinates, you get a positive value. But wait. If you divide those differences, you get the slope of line k. So Plug (a, b) and (r, s) into the slope formula: slope = (b − s) ÷ (a − r)
If (a − r)(b − s) > 0, then either both differences are positive or both are negative. And if (a − r) and (b − s) yield differences with matching signs, then (b − s) ÷ (a − r) > 0, meaning the slope of line k must be positive. So if C is true, then the slope of line k must be positive.
A, B, and C are all correct.









