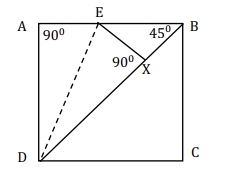
Let us focus on triangle ADB.
We know that DE is the internal angle bisector of ∠ADB.
In any equilateral triangle, the angle bisector also bisects the side opposite to the angle
it bisects.
Also, in an isosceles triangle, the angle bisector of the ‘unequal angle’ also bisects the
side opposite to that angle, i.e. it bisects the ‘unequal side’.
In this case, though triangle ADB is isosceles, the angle bisector bisects one of the equal angles
(since ∠ADB = ∠ABD = 45◦).
Thus, the angle bisector in this case, i.e. DE, does not bisect the side opposite to the
concerned angle.
Thus, DE does not bisect AB
=> AE 6= BE
Let us draw EX perpendicular to BD.





It is clear that triangles ADE and XDE are congruent to one another, since
DE is a common side, ∠DAE =∠DXE = 90◦, and ∠ADE =∠XDE
Thus, AE = EX.
In triangle EXB, EB is the hypotenuse, and hence, the longest side.
Thus, we have
EB > EX
=> EB > AE
Thus, AE must be less than half of AB
=> AE < 0.5