Solution

Here we have two circles with centers A and B they intersect on points say X and Y. Now to find the area of the shaded region.

Let us connect the points X, A, B to form a triangle and Y, A, B to form another triangle. Since the radius of circle A and B are same. We can say that XAB is an equilateral triangle. With XA=AB= XB= radius of circle. Similarly YAB is also a triangle.

So now we can clearly see that the area of the two triangles = $2*\sqrt{3}*\frac{x^2}{4}$. ........(i)


Now the segment, i.e. the area between the triangle and a circle.



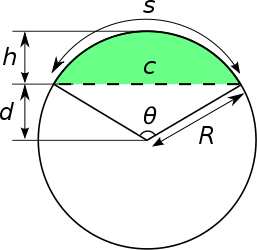
Area of segment = $\frac{x^2}{2}(\frac{pi*theta}{180}- sin(theta))$.

Where theta is the central angle.
Or in our case angle XAB = 60 degrees= theta.

Putting theta = 60 we have

Area of segment= $\frac{pi*x^2}{6} - \sqrt{3}\frac{x^2}{2}$.

Now we have 4 such segments. Now adding this to (i) and simplifying we get

Area of the shaded region as = $\frac{4*pi}{6}*x^2 - 3*\sqrt{3}\frac{1}{2}*x^2$