GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
The table below provides values of quadratic function f for some value
[#permalink]
23 Feb 2022, 05:45
23 Feb 2022, 05:45
Expert Reply
1
Bookmarks
00:00
Question Stats:
75% (01:08) correct
25% (01:10) wrong based on 16 sessions
Hide Show timer Statistics
The table below provides values of quadratic function f for some values x. Which of the following equations could define f(x)?
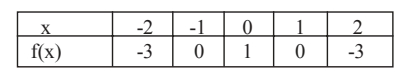
GRe The table below.jpg [ 12.83 KiB | Viewed 1768 times ]
(A) \(f(x) = x^2 + x - 1 \)
(B) \(f(x) = x^2 - 1\)
(C) \(f(x) = -x^2 + x + 1 \)
(D) \(f(x) = -x^2 + 1\)
(E) \(f(x) = x^2 - x + 1 \)
Attachment:
GRe The table below.jpg [ 12.83 KiB | Viewed 1768 times ]
(A) \(f(x) = x^2 + x - 1 \)
(B) \(f(x) = x^2 - 1\)
(C) \(f(x) = -x^2 + x + 1 \)
(D) \(f(x) = -x^2 + 1\)
(E) \(f(x) = x^2 - x + 1 \)
Re: The table below provides values of quadratic function f for some value
[#permalink]
23 Feb 2022, 10:47
23 Feb 2022, 10:47
Carcass wrote:
The table below provides values of quadratic function f for some values x. Which of the following equations could define f(x)?
(A) \(f(x) = x^2 + x - 1 \)
(B) \(f(x) = x^2 - 1\)
(C) \(f(x) = -x^2 + x + 1 \)
(D) \(f(x) = -x^2 + 1\)
(E) \(f(x) = x^2 - x + 1 \)
Attachment:
GRe The table below.jpg
(A) \(f(x) = x^2 + x - 1 \)
(B) \(f(x) = x^2 - 1\)
(C) \(f(x) = -x^2 + x + 1 \)
(D) \(f(x) = -x^2 + 1\)
(E) \(f(x) = x^2 - x + 1 \)
I found this question a little lengthy as I have to pick and choose the numbers to test all equations. Is there a shortcut or strategy to pick no. smartly.
Retired Moderator
Joined: 10 Apr 2015
Posts: 6218
Given Kudos: 136
Re: The table below provides values of quadratic function f for some value
[#permalink]
24 Feb 2022, 05:59
24 Feb 2022, 05:59
1
Carcass wrote:
The table below provides values of quadratic function f for some values x. Which of the following equations could define f(x)?
(A) \(f(x) = x^2 + x - 1 \)
(B) \(f(x) = x^2 - 1\)
(C) \(f(x) = -x^2 + x + 1 \)
(D) \(f(x) = -x^2 + 1\)
(E) \(f(x) = x^2 - x + 1 \)
Attachment:
GRe The table below.jpg
(A) \(f(x) = x^2 + x - 1 \)
(B) \(f(x) = x^2 - 1\)
(C) \(f(x) = -x^2 + x + 1 \)
(D) \(f(x) = -x^2 + 1\)
(E) \(f(x) = x^2 - x + 1 \)
Let's eliminate answers choices by plugging easy-to-work-with numbers into each function.
For example, the table tells us that f(0) = 1, so let's plug x = 0 into each function and eliminate those that don't evaluate to 1.
We get:
(A) \(f(0) = 0^2 + 0 - 1 =-1\). Eliminate
(B) \(f(0) = 0^2 - 1 = -1\). Eliminate
(C) \(f(0) = -(0^2) + 0 + 1 = 1\). Keep
(D) \(f(0) = -(0^2) + 1 = 1\). Keep
(E) \(f(0) = 0^2 - 0 + 1 = 1\). Keep
The table also tells us that, when f(1) = 0, so let's plug x = 1 into each remaining function and eliminate those that don't evaluate to 0.
We get:
(C) \(f(1) = -(1^2) + 1 + 1 = 1 \). Eliminate
(D) \(f(1) = -(1^2) + 1 = 0\). Keep
(E) \(f(1) = 1^2 - 1 + 1 = 1\). Eliminate
By the process of elimination, the correct answer is D
