
GRE Prep Club Daily Prep
Thank you for using the timer - this advanced tool can estimate your performance and suggest more practice questions. We have subscribed you to Daily Prep Questions via email.
Customized
for You
Track
Your Progress
Practice
Pays
Not interested in getting valuable practice questions and articles delivered to your email? No problem, unsubscribe here.
GRE Math Essentials for a TOP Quant Score - Q170!!!
[#permalink]
 19 Jun 2021, 13:37
19 Jun 2021, 13:37
3
Expert Reply
6
Bookmarks
The GRE Math Essentials project is the best complement to our Official GRE Quant Book. It provides a cutting-edge, in-depth overview of all the math concepts to achieve 170 in the Quantitative Reasoning portion of the GRE. Our book still remains our hallmark. However, the following chapters will give you a lot of tips, tricks, and shortcuts to make your quant preparation more robust and solid.
According to our estimation, the algebra portion of the GRE test, the frequency of the algebra questions tested, is about 20 to 23 %; in numbers, 4 to 6 questions, you have to deal with, are based on the Algebraic topic.
On one extreme of the spectrum, we need, to score well in that quantitative portion of the test, firm quant foundations. On the other extreme of the spectrum, we need to practice good questions until our mental quant process is rock solid. i.e., when we have to solve a question, the approach to it, the way we went through the same process is basically on an automatic pilot.
Thanks to rx10 contributed to!!!
reference: A maths dictionary for kids - a quick reference + maths charts in PDF by Jenny Eather
- Numbers Comprehension
- Exponents
- Common Binomial Expressions
- Aggregate of Series
- Fractions
- Ratios
- Percent %
- Words into math
- Interest
- Expressions
- Equations
- Absolute Numbers
- Functions
Show: ::
Attachment:
algebra tip.png [ 88.99 KiB | Viewed 7739 times ]
Attachment:
screenshot.83.png [ 145.62 KiB | Viewed 38329 times ]
Attachment:
gre fundamentals quant.png [ 16.51 KiB | Viewed 113250 times ]
Attachment:
GRE Fundamentals.jpg [ 72.77 KiB | Viewed 5503 times ]
Attachment:
GRE Prep Club.png [ 20.89 KiB | Viewed 1304 times ]
Re: GRE Math Essentials for a TOP Quant Score - Q170!!!
[#permalink]
 15 Sep 2021, 12:29
15 Sep 2021, 12:29
1
Expert Reply
1) Types of numbers
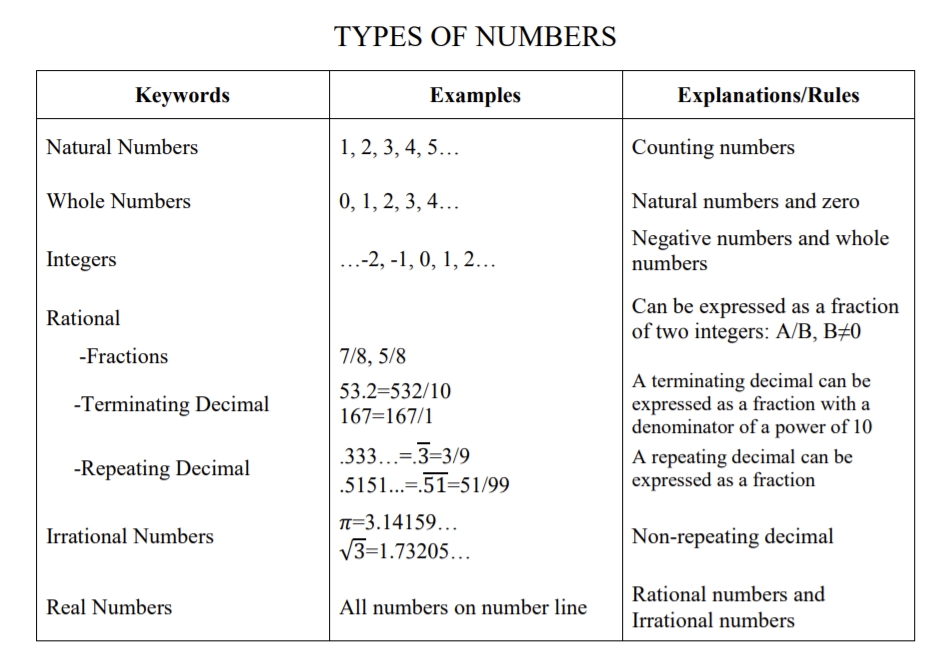
Note \(\sqrt{negative numbers}\) or \(\frac{number}{0-zero}\) are NOT real !!
On the GRE by default, any number mentioned in the quant section is a real number so must NOT assume that it is an integer unless stated clearly
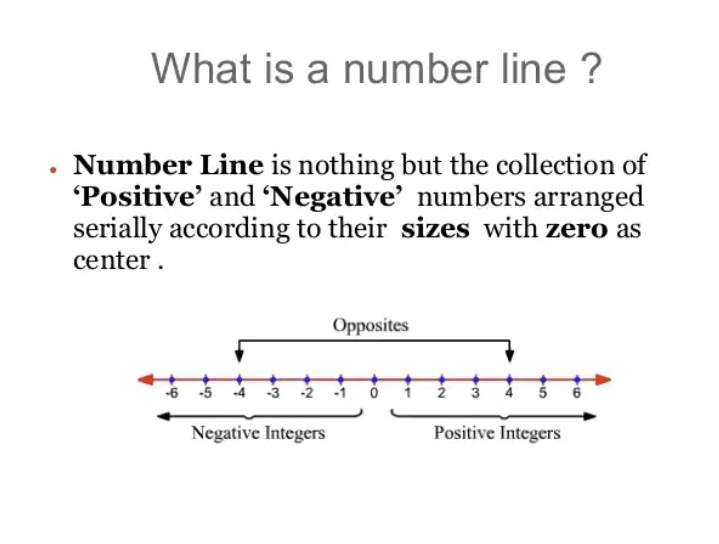
2) Number Line
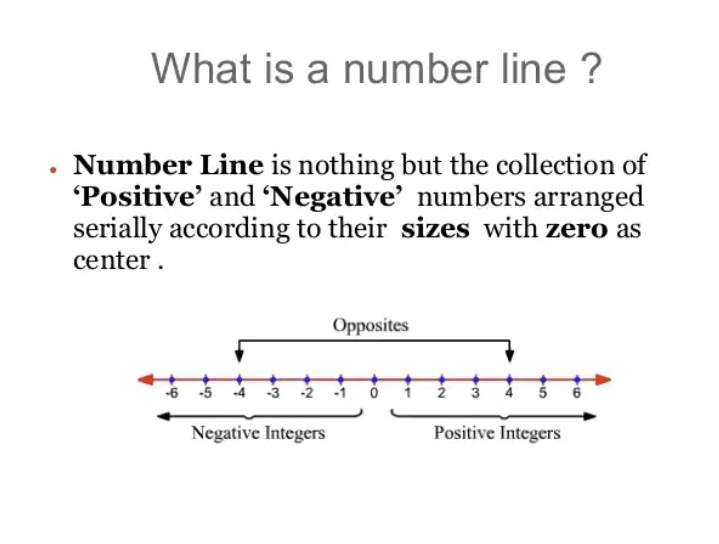
3) Basic operations on numbers
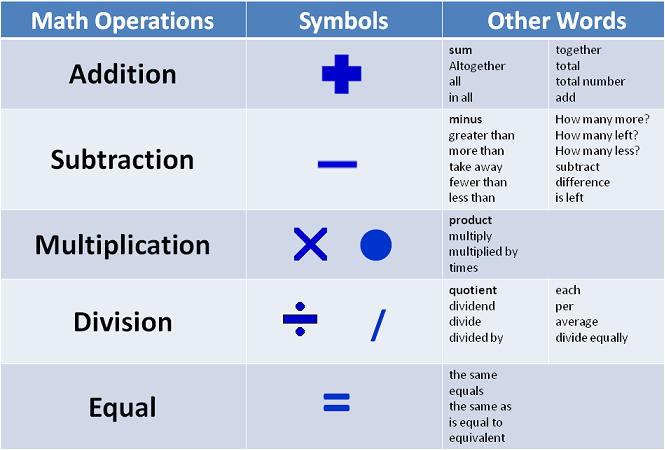
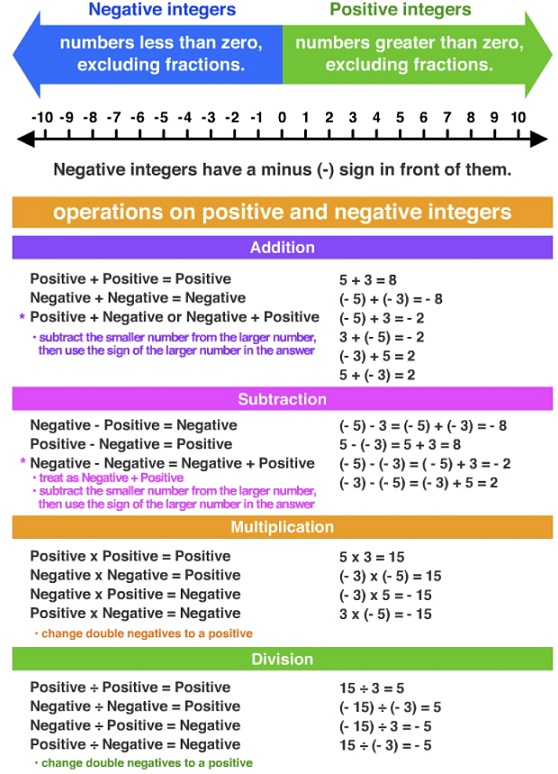
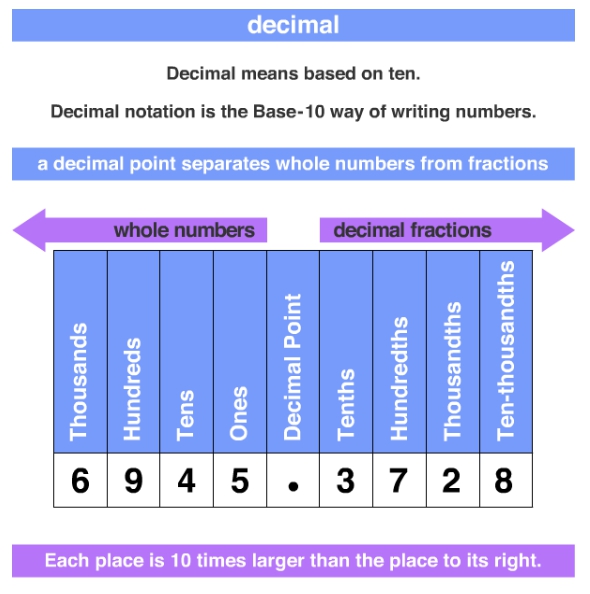
4) Place and Face Value
5) Basic operation on Even and Odd Numbers
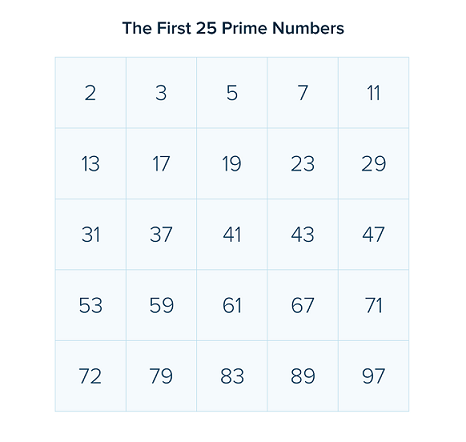
6) Prime Numbers
A number greater than 1 which has no factor other than 1 and the number itself is a Prime Number
A number with two distinct factors.
We do have infinite prime numbers and apparently, to our knowledge today, there is NO clear pattern in their unfolding toward the infinite on the number line.
Prime numbers are only positive.
Two (2) is the oNLy positive Even prime numbers
The difference between any two primes greater than 2 is always even.
Other than 2 and 3, if divided by 6, will always leave a remainder of 1 or 5. Any prime cab be written or expressed as \(6k+1\) where k is some positive integer.
Not all numbers, however, which leave a remainder of 1 or 5 when divided by 6 are prime such as 25,35.
Verifying if a number integer and positive is a prime, regardless
1) Take square root of the number, if it is an integer then the number is NOT a prime number;
2) Consider the largest integer closest to the square root of the number n is m , where \(m < \sqrt{n}\)
3) Divide n by all the prime numbers from \(2 to m\), inclusive: if n is divisible by any number among them, the number n is NOT a prime, else is a prime.
\(n=101\)
\(10 < \sqrt{101} <11\) is not an integer
\(101 = \approx 10=m\)
Divide 101 by prime numbers 2 to 10 or 2,3,5,7. Since 101 is not divisible by any prime number listed then 101 MUST be a prime number.
7) Rounding

Rounding Rules
more than 5 > up
less than 5 < down
~ for decimal fractions
1. Look at the digit in the place value to be rounded to.
2. Increase it by 1 if the digit to the right of it is 5 or more.
3. Leave it the same if the digit to the right of it is less than 5.
4. Remove everything to the right of the digit.
Example:
Round 625.475 to 1 decimal place: 625.475 ≈ 625.5
~ when rounding to 10 or above there's an important change to step 4.
4. Replace whole numbers to the right of the digit with zero(s), then remove everything to their right.
Examples:
Round 625.475 to 10: 625.475 ≈ 630
Round 625.475 to 100: 625.475 ≈ 600
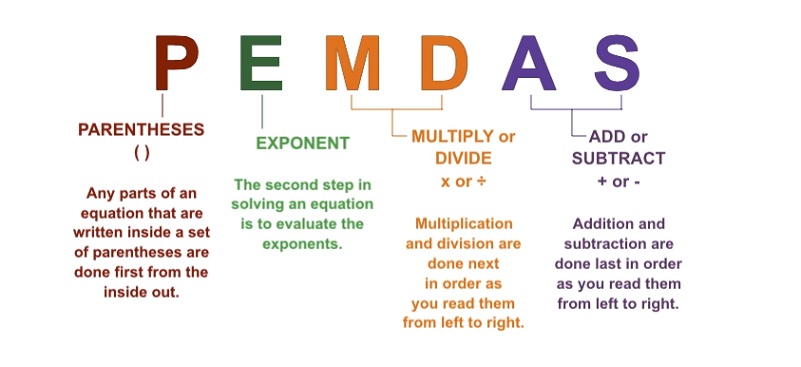
8) P.E.M.D.A.S
9) Divisibility Rules
10) Factors
Factor
• a whole number that divides exactly into another number.
• a whole number that multiplies with another number to make a third number.
Factors Pair
• a pair of numbers multiplied together form another number called their product.

Number of factors
A number N can be written as \( N=a^xb^yc^z\), where a,b,and c ar prime factors of the number N, and x,y, and z are positive integers.
Number of factors : (including 1 and the number itself)\(=(x+1)(y+1)(z+1)\);
Factors of \(24=2*2*2*3=2^3*3^1\)
The exponent are 3 and 1 ====> \((3+1)(1+1)=2*4=8\)
Sum of all the factors
A number N can be written as \( N=a^xb^yc^z\), where a,b,and c ar prime factors of the number N, and x,y, and z are positive integers.
Sum of all the factors (including 1 and the number itself)
\(\left[ \begin{array}{cc|r} \frac{a^{x+1}-1}{a-1} \end{array} \right]\) \(\left[ \begin{array}{cc|r} \frac{b^{y+1}-1}{b-1} \end{array} \right]\) \(\left[ \begin{array}{cc|r} \frac{c^{z+1}-1}{c-1} \end{array} \right]\)
11) Perfect Square Numbers
A perfect square number is a non-negative integer that can be expressed as the product of an integer with itself. I.E., a number that is a square of any integer is called a Perfect Square number. Perfect square numbers are always non-negative.
Some properties of a perfect square:
Example
Of the following, which one is a perfect square?
a) 126736
b) 137826
c) 149913
The only number that has both of the combinations at the same time is a) 126736
The unit digit is 6, AND the tenth digit is ODD
Question to practice about perfect square
https://gre.myprepclub.com/forum/if-a-perf ... tml#p59618
https://gre.myprepclub.com/forum/a-perfect ... tml#p32573
https://gre.myprepclub.com/forum/positive- ... tml#p39436
https://gre.myprepclub.com/forum/a-perfect ... tml#p51029
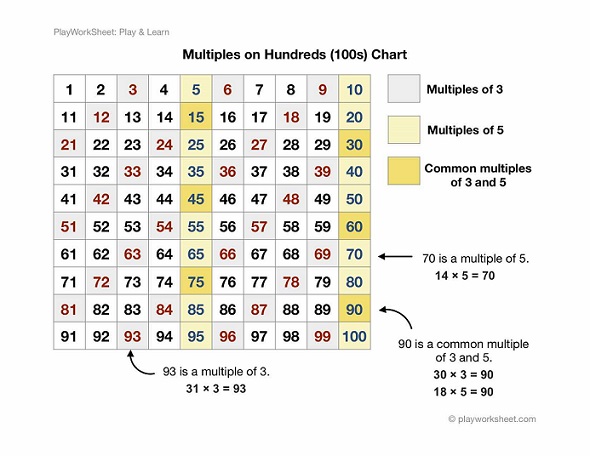
12) Multiples
13) HCF (GCD / GCF) & LCM OF NUMBERS
HCF : It is the greatest factor common to two or more given numbers. It is also called GCF OR GCD (greatest common factor or greatest common divisor). e.g. HCF of 10 & 15 = 5, HCF of 55 & 200 = 5, HCF of 64 & 36 = 4
To find the HCF of given numbers, resolve the numbers into their prime factors and then pick the common term(s) from them and multiply them if more than one. This is the required HCF.
LCM : Lowest common multiple of two or more numbers is the smallest number which is exactly divisible by all of them. e.g. LCM of 5, 7, 10 = 70, LCM of 2, 4, 5 = 20, LCM of 11, 10, 3 = 330
To find the LCM resolve all the numbers into their prime factors and then pick all the quantities (prime factors) but not more than once and multiply them. This is the LCM.
NOTE:
1. LCM x HCF = Product of two numbers (valid only for “two”)
2. HCF of fractions = HCF of numerators ÷ LCM of denominators
3. LCM of fractions = LCM of numerators ÷ HCF of denominators
Calculating LCM :
Method 1 : Factorization Method
Rule – After expressing the numbers in terms of prime factors, the LCM is the product of highest powers of all factors.
Q. Find the LCM of 40, 120, 380.
A. 4\(0 = 4 \times 10 = 2 \times 2 \times 2 \times 5 = 2^3 \times 5^1\)
\(120 = 4 \times 30 = 2 \times 2 \times 2 \times 5 \times 3 = 2^3 \times 5^1 \times 3^1\)
\(380 = 2 \times 190 = 2 \times 2 \times 95 = 2 \times 2 \times 5 \times 19 = 2^2 \times 5^1 \times 19^1\)
⇒ Required LCM = \(2^3 \times 5^1 \times 3^1 \times 19^1 = 2280\).
Method 2 : Division method
Q. Find the LCM of 6, 10, 15, 24, 39
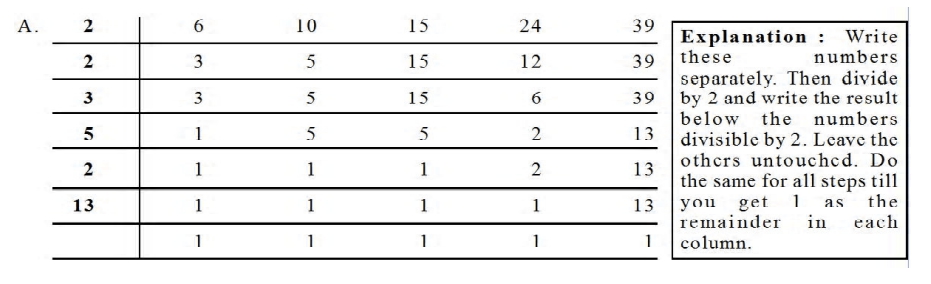
Ans. LCM = 2 x 2 x 3 x 5 x 2 x 13 = 1560.
Calculating HCF
Factorization
After expressing the numbers in term of the prime factors, the HCF is product of COMMON factors.
Ex. Find HCF of 88, 24, 124
\(88 = 2 \times 44 = 2 \times 2 \times 22 = 2 \times 2 \times 2 \times 11 = 2^3 \times 11^1\)
\(24 = 2 \times 12 = 2 \times 2 \times 6 = 2 \times 2 \times 2 \times 3 = 2^3 \times 3^1\)
\(124 = 2 \times 62 = 2 \times 2^1 \times 31^1 = 2^2 \times 31^1 ⇒ HCF = 2^2\)

GRE Types of Numbers.jpg [ 232.54 KiB | Viewed 5267 times ]
GRE Number Line.jpg [ 91.59 KiB | Viewed 5247 times ]
GRE basic operations with numbers.png [ 75.59 KiB | Viewed 5218 times ]

GRE Face and Place Value.jpg [ 141.16 KiB | Viewed 5229 times ]
GRE basic operations with numbers.jpg [ 155.48 KiB | Viewed 5233 times ]
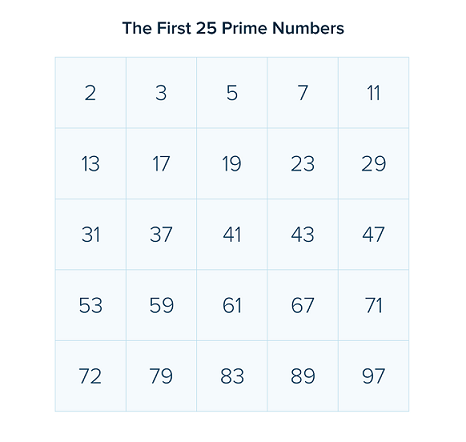
first_25_prime_numbers.png [ 39.61 KiB | Viewed 4449 times ]
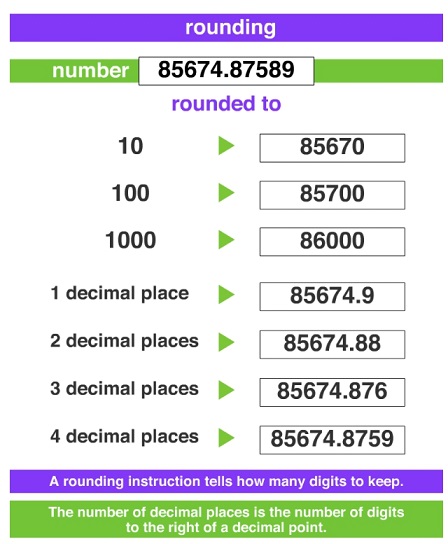
GRE rounding.jpg [ 66.47 KiB | Viewed 4436 times ]
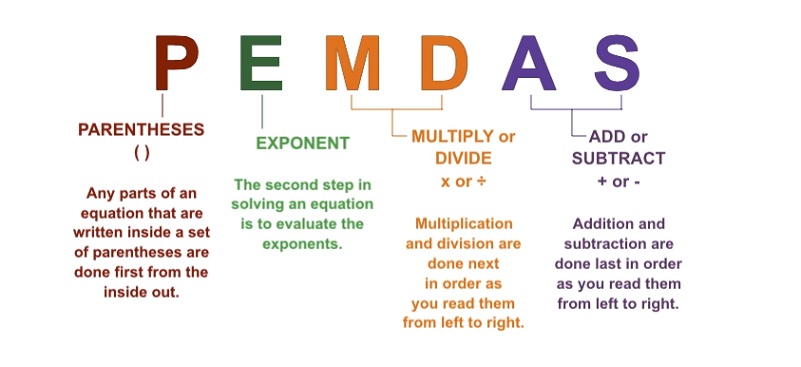
GRE Pemdas.jpg [ 58.79 KiB | Viewed 4431 times ]
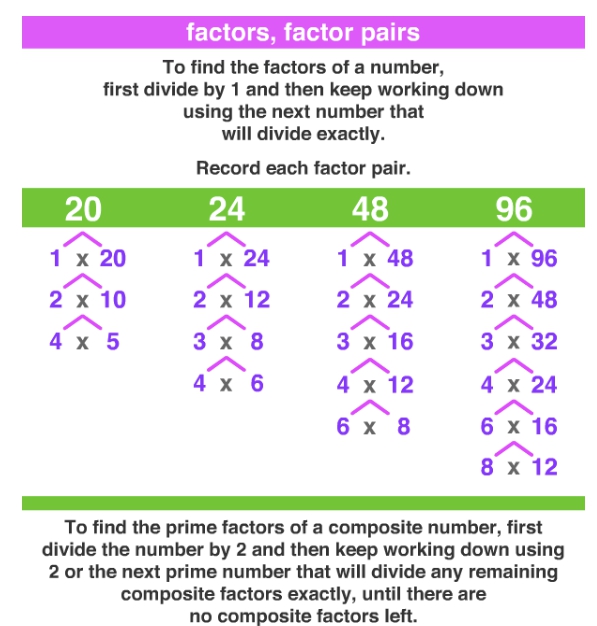
GRE factors.jpg [ 166.07 KiB | Viewed 3764 times ]
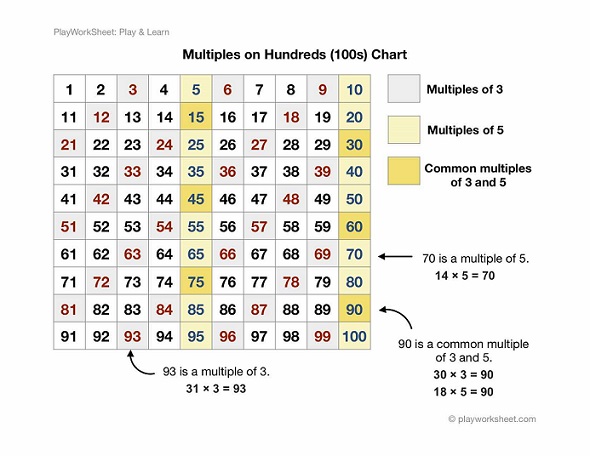
multiples-hundreds-chart.jpg [ 77.83 KiB | Viewed 3749 times ]
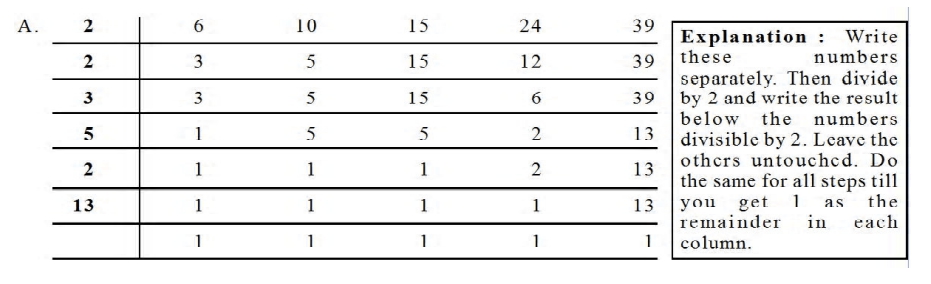
GRE LCM.jpg [ 120.65 KiB | Viewed 3732 times ]
Note \(\sqrt{negative numbers}\) or \(\frac{number}{0-zero}\) are NOT real !!
On the GRE by default, any number mentioned in the quant section is a real number so must NOT assume that it is an integer unless stated clearly
2) Number Line
3) Basic operations on numbers
4) Place and Face Value
| Place Value | Face Value |
| Place value is the value represented by a digit in a number according to its position in the number. | Face value is the actual value of a digit in a number. |
| To get the place value of a number, we multiply the digit value with its numerical value. For example, in the number 452, the place value of 5 is (5 × 10) = 50, since 5 is in tens place. | The face value of a digit is the number itself. For example, in the number 452, the face value of 4 is 4. |
| The place value of a number depends upon the position of the digit in the number. | The face value is independent of the position of the digit in the number. |
| The place value of a digit in ones place is always a single digit and the place value of every next digit to the left increases by one more digit. | The face value of a number is always a single digit. |
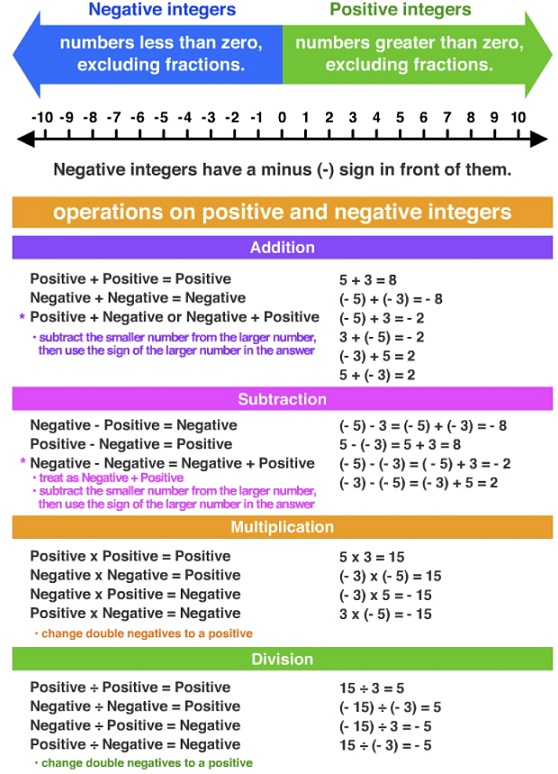
5) Basic operation on Even and Odd Numbers
| Basic operation on Even and Odd Numbers | |||||
Math operations | Application | Examples | |||
| Addition/Subtraction | Even \(\pm\) Even = Even; Odd \(\pm\) Odd = Even; Even \(\pm\) Odd = Odd Any number of even numbers can make an even number; but only an even number of odd numbers can make an even number. |
| |||
| Multiplication | Even \(\times\) Even = Even; Odd \(\times\) Odd = Odd; Even \(\times\) Odd = Even Multiplication of any number of odd numbers always results in another odd number. If the product of two integers is Even and one of them is an Even integer, we cannot assess on the nature of the other number; i.e. if it is Even or Odd | 4 \(\times\) 2=8; Even; 3 \(\times\) 3 = 9; Odd; 2 \(\times\) 3 = 6; Even | |||
| Division | Not every operation of Division is completely divisible. The division could leave remainder or not Odd / Odd = Odd; Even / Odd = Even; Odd / Even = Not divisible; Even / Even = Even or Odd | 9/3=3; Odd 10/5=2; Even 11/2=? Not divisible 4/2=2; Even 10/2=5; Odd | |||
6) Prime Numbers
A number greater than 1 which has no factor other than 1 and the number itself is a Prime Number
A number with two distinct factors.
We do have infinite prime numbers and apparently, to our knowledge today, there is NO clear pattern in their unfolding toward the infinite on the number line.
Prime numbers are only positive.
Two (2) is the oNLy positive Even prime numbers
The difference between any two primes greater than 2 is always even.
Other than 2 and 3, if divided by 6, will always leave a remainder of 1 or 5. Any prime cab be written or expressed as \(6k+1\) where k is some positive integer.
Not all numbers, however, which leave a remainder of 1 or 5 when divided by 6 are prime such as 25,35.
Verifying if a number integer and positive is a prime, regardless
1) Take square root of the number, if it is an integer then the number is NOT a prime number;
2) Consider the largest integer closest to the square root of the number n is m , where \(m < \sqrt{n}\)
3) Divide n by all the prime numbers from \(2 to m\), inclusive: if n is divisible by any number among them, the number n is NOT a prime, else is a prime.
\(n=101\)
\(10 < \sqrt{101} <11\) is not an integer
\(101 = \approx 10=m\)
Divide 101 by prime numbers 2 to 10 or 2,3,5,7. Since 101 is not divisible by any prime number listed then 101 MUST be a prime number.
7) Rounding
Rounding Rules
more than 5 > up
less than 5 < down
~ for decimal fractions
1. Look at the digit in the place value to be rounded to.
2. Increase it by 1 if the digit to the right of it is 5 or more.
3. Leave it the same if the digit to the right of it is less than 5.
4. Remove everything to the right of the digit.
Example:
Round 625.475 to 1 decimal place: 625.475 ≈ 625.5
~ when rounding to 10 or above there's an important change to step 4.
4. Replace whole numbers to the right of the digit with zero(s), then remove everything to their right.
Examples:
Round 625.475 to 10: 625.475 ≈ 630
Round 625.475 to 100: 625.475 ≈ 600
8) P.E.M.D.A.S
9) Divisibility Rules
1. A number is divisible by 2 if its unit’s digit is even or zero e.g. 128, 146, 34 etc.
2. A number is divisible by 3 if the sum of its digits is divisible by 3 e.g. 102, 192, 99 etc.
3. A number is divisible by 4 when the number formed by last two right hand digits is divisible by ‘4’ e.g. 576, 328, 144 etc.
4. A number is divisible by 5 when its unit’s digit is either five or zero : e.g. 1111535, 3970, 145 etc.
5. A number is divisible by 6 when it’s divisible by 2 and 3 both. e.g. 714, 509796, 1728 etc.
6. A number is divisible by 8 when the number formed by the last three right hand digits is divisible by ‘8’. e.g. 512, 4096, 1304 etc.
7. A number is divisible by 9 when the sum of its digits is divisible by 9 e.g. 1287, 11583, 2304 etc.
8. A number is divisible by 10 when its units digit is zero. e.g. 100, 170, 10590 etc.
9. A number is divisible by 11 when the difference between the sums of digits in the odd and even places is either zero or a multiple of 11. e.g. 17259, 62468252, 12221 etc.
For the number 17259 : Sum of digits in even places = 7 + 5 = 12, Sum of digits in the odd places = 1 + 2 + 9 =12 Hence 12 – 12 = 0.
10. A number is divisible by 12 when it is divisible by 3 & 4 both. e.g. 672, 8064 etc.
11. A number is divisible by 25 when the number formed by the last two Right hand digits is divisible by 25. e.g. 1025, 3475, 55550 etc.
12. A number is divisible by 125, when the number formed by last three right hand digits is divisible by 125. e.g. 2125, 4250, 6375 etc.
NOTE :
1. When any number with even number of digits is added to its reverse, the sum is always divisible by 11. e.g. 2341 + 1432 = 3773 which is divisible by 11.
2. If X is a prime number then for any whole number “a” \((a^X – a)\) is divisible by X e.g. Let X = 3 and a = 5. Then according to our rule \(5^3– 5\) should be divisible by 3.
Now \((5^3– 5) = 120\) which is divisible by 3
2. A number is divisible by 3 if the sum of its digits is divisible by 3 e.g. 102, 192, 99 etc.
3. A number is divisible by 4 when the number formed by last two right hand digits is divisible by ‘4’ e.g. 576, 328, 144 etc.
4. A number is divisible by 5 when its unit’s digit is either five or zero : e.g. 1111535, 3970, 145 etc.
5. A number is divisible by 6 when it’s divisible by 2 and 3 both. e.g. 714, 509796, 1728 etc.
6. A number is divisible by 8 when the number formed by the last three right hand digits is divisible by ‘8’. e.g. 512, 4096, 1304 etc.
7. A number is divisible by 9 when the sum of its digits is divisible by 9 e.g. 1287, 11583, 2304 etc.
8. A number is divisible by 10 when its units digit is zero. e.g. 100, 170, 10590 etc.
9. A number is divisible by 11 when the difference between the sums of digits in the odd and even places is either zero or a multiple of 11. e.g. 17259, 62468252, 12221 etc.
For the number 17259 : Sum of digits in even places = 7 + 5 = 12, Sum of digits in the odd places = 1 + 2 + 9 =12 Hence 12 – 12 = 0.
10. A number is divisible by 12 when it is divisible by 3 & 4 both. e.g. 672, 8064 etc.
11. A number is divisible by 25 when the number formed by the last two Right hand digits is divisible by 25. e.g. 1025, 3475, 55550 etc.
12. A number is divisible by 125, when the number formed by last three right hand digits is divisible by 125. e.g. 2125, 4250, 6375 etc.
NOTE :
1. When any number with even number of digits is added to its reverse, the sum is always divisible by 11. e.g. 2341 + 1432 = 3773 which is divisible by 11.
2. If X is a prime number then for any whole number “a” \((a^X – a)\) is divisible by X e.g. Let X = 3 and a = 5. Then according to our rule \(5^3– 5\) should be divisible by 3.
Now \((5^3– 5) = 120\) which is divisible by 3
10) Factors
Factor
• a whole number that divides exactly into another number.
• a whole number that multiplies with another number to make a third number.
Factors Pair
• a pair of numbers multiplied together form another number called their product.
Number of factors
A number N can be written as \( N=a^xb^yc^z\), where a,b,and c ar prime factors of the number N, and x,y, and z are positive integers.
Number of factors : (including 1 and the number itself)\(=(x+1)(y+1)(z+1)\);
Factors of \(24=2*2*2*3=2^3*3^1\)
The exponent are 3 and 1 ====> \((3+1)(1+1)=2*4=8\)
Sum of all the factors
A number N can be written as \( N=a^xb^yc^z\), where a,b,and c ar prime factors of the number N, and x,y, and z are positive integers.
Sum of all the factors (including 1 and the number itself)
\(\left[ \begin{array}{cc|r} \frac{a^{x+1}-1}{a-1} \end{array} \right]\) \(\left[ \begin{array}{cc|r} \frac{b^{y+1}-1}{b-1} \end{array} \right]\) \(\left[ \begin{array}{cc|r} \frac{c^{z+1}-1}{c-1} \end{array} \right]\)
11) Perfect Square Numbers
A perfect square number is a non-negative integer that can be expressed as the product of an integer with itself. I.E., a number that is a square of any integer is called a Perfect Square number. Perfect square numbers are always non-negative.
| Unit's digit | 0 | 1 | 4 | 5 | 6 | 9 |
| Ten's digit | 0 | even | even | 2 | odd | even |
Some properties of a perfect square:
- Perfcet square number ALWAYS has even number of powers of prime factors;
- The number of distinct factors of a perfect square number is ALWAYS odd. (By factors we refer to only the "positive numbers that divide the number withouth remainder);
- The sum of distinct factors of a perfect square number is ALWAYS odd;
- A percet square number ALWAYS has an odd number of odd-factors, and even number of even-factors;
Example
Of the following, which one is a perfect square?
a) 126736
b) 137826
c) 149913
The only number that has both of the combinations at the same time is a) 126736
The unit digit is 6, AND the tenth digit is ODD
Question to practice about perfect square
https://gre.myprepclub.com/forum/if-a-perf ... tml#p59618
https://gre.myprepclub.com/forum/a-perfect ... tml#p32573
https://gre.myprepclub.com/forum/positive- ... tml#p39436
https://gre.myprepclub.com/forum/a-perfect ... tml#p51029
12) Multiples
13) HCF (GCD / GCF) & LCM OF NUMBERS
HCF : It is the greatest factor common to two or more given numbers. It is also called GCF OR GCD (greatest common factor or greatest common divisor). e.g. HCF of 10 & 15 = 5, HCF of 55 & 200 = 5, HCF of 64 & 36 = 4
To find the HCF of given numbers, resolve the numbers into their prime factors and then pick the common term(s) from them and multiply them if more than one. This is the required HCF.
LCM : Lowest common multiple of two or more numbers is the smallest number which is exactly divisible by all of them. e.g. LCM of 5, 7, 10 = 70, LCM of 2, 4, 5 = 20, LCM of 11, 10, 3 = 330
To find the LCM resolve all the numbers into their prime factors and then pick all the quantities (prime factors) but not more than once and multiply them. This is the LCM.
NOTE:
1. LCM x HCF = Product of two numbers (valid only for “two”)
2. HCF of fractions = HCF of numerators ÷ LCM of denominators
3. LCM of fractions = LCM of numerators ÷ HCF of denominators
Calculating LCM :
Method 1 : Factorization Method
Rule – After expressing the numbers in terms of prime factors, the LCM is the product of highest powers of all factors.
Q. Find the LCM of 40, 120, 380.
A. 4\(0 = 4 \times 10 = 2 \times 2 \times 2 \times 5 = 2^3 \times 5^1\)
\(120 = 4 \times 30 = 2 \times 2 \times 2 \times 5 \times 3 = 2^3 \times 5^1 \times 3^1\)
\(380 = 2 \times 190 = 2 \times 2 \times 95 = 2 \times 2 \times 5 \times 19 = 2^2 \times 5^1 \times 19^1\)
⇒ Required LCM = \(2^3 \times 5^1 \times 3^1 \times 19^1 = 2280\).
Method 2 : Division method
Q. Find the LCM of 6, 10, 15, 24, 39
Ans. LCM = 2 x 2 x 3 x 5 x 2 x 13 = 1560.
Calculating HCF
Factorization
After expressing the numbers in term of the prime factors, the HCF is product of COMMON factors.
Ex. Find HCF of 88, 24, 124
\(88 = 2 \times 44 = 2 \times 2 \times 22 = 2 \times 2 \times 2 \times 11 = 2^3 \times 11^1\)
\(24 = 2 \times 12 = 2 \times 2 \times 6 = 2 \times 2 \times 2 \times 3 = 2^3 \times 3^1\)
\(124 = 2 \times 62 = 2 \times 2^1 \times 31^1 = 2^2 \times 31^1 ⇒ HCF = 2^2\)
Show: ::
Attachment:
GRE Types of Numbers.jpg [ 232.54 KiB | Viewed 5267 times ]
Attachment:
GRE Number Line.jpg [ 91.59 KiB | Viewed 5247 times ]
Attachment:
GRE basic operations with numbers.png [ 75.59 KiB | Viewed 5218 times ]
Attachment:
GRE Face and Place Value.jpg [ 141.16 KiB | Viewed 5229 times ]
Attachment:
GRE basic operations with numbers.jpg [ 155.48 KiB | Viewed 5233 times ]
Attachment:
first_25_prime_numbers.png [ 39.61 KiB | Viewed 4449 times ]
Attachment:
GRE rounding.jpg [ 66.47 KiB | Viewed 4436 times ]
Attachment:
GRE Pemdas.jpg [ 58.79 KiB | Viewed 4431 times ]
Attachment:
GRE factors.jpg [ 166.07 KiB | Viewed 3764 times ]
Attachment:
multiples-hundreds-chart.jpg [ 77.83 KiB | Viewed 3749 times ]
Attachment:
GRE LCM.jpg [ 120.65 KiB | Viewed 3732 times ]
Re: GRE Math Essentials for a TOP Quant Score - Q170!!!
[#permalink]
 15 Sep 2021, 12:30
15 Sep 2021, 12:30
Expert Reply
1) Exponents
Note \((a^m)^n \neq a^{m^n}\)
\((2^2)^3 = 2^6=64\)
\(2^{3^2}=2^9=512 \neq 64\)
2) Unit Digit Power
Example
Find the unit digit of \(7^{99}\)?
Having a cyclicity of 4 we do have that \(7^{10}\) has a unit digit of 9. This for nine times which is \(7^{90} =\) unit digit of 9 + 9 spots the unit digit is 3
Question to practice about perfect square
https://gre.myprepclub.com/forum/p-23-25-2 ... tml#p43949
https://gre.myprepclub.com/forum/if-x-is-a ... tml#p32890
https://gre.myprepclub.com/forum/qotd-7-n- ... html#p5177
https://gre.myprepclub.com/forum/y-20504.html#p60545
https://gre.myprepclub.com/forum/for-which ... tml#p42721
https://gre.myprepclub.com/forum/units-dig ... tml#p62633
3) Unit Digit of a Square
The square of a number can never end with \(2, 3, 7, 8\) or odd number of zeros.
4) Number rearrangement
When a two-digit number is reversed then the sum of two numbers is always divisible by 11 & the difference of two numbers is always divisible by 9
Let's say we have a number 92
When reversed it will be 29
Sum = 92+29 = \(121\) ----> Divisible by \(11\)
Difference = 92-29 = \(63\) ----> Divisible by \(9\)
5) Square of 5
Suppose that X is a number ending in 5 or 5 itself
\((X5)^2=X(X+1),25\)
\(625^2=62 \times 63\),\(25\)\(=\)\(3906\)\(25\)
You just need to multiply \(62 \times 63\) and add \(25\)
6) Square of any number
\(n^2=(n-d)(n+d)+d^2\)
d is the distance from the nearest multiple of 10.100
\(19^2=(19-1)(19+1)+1^2=18 \times 20+1=361\)
2) Roots
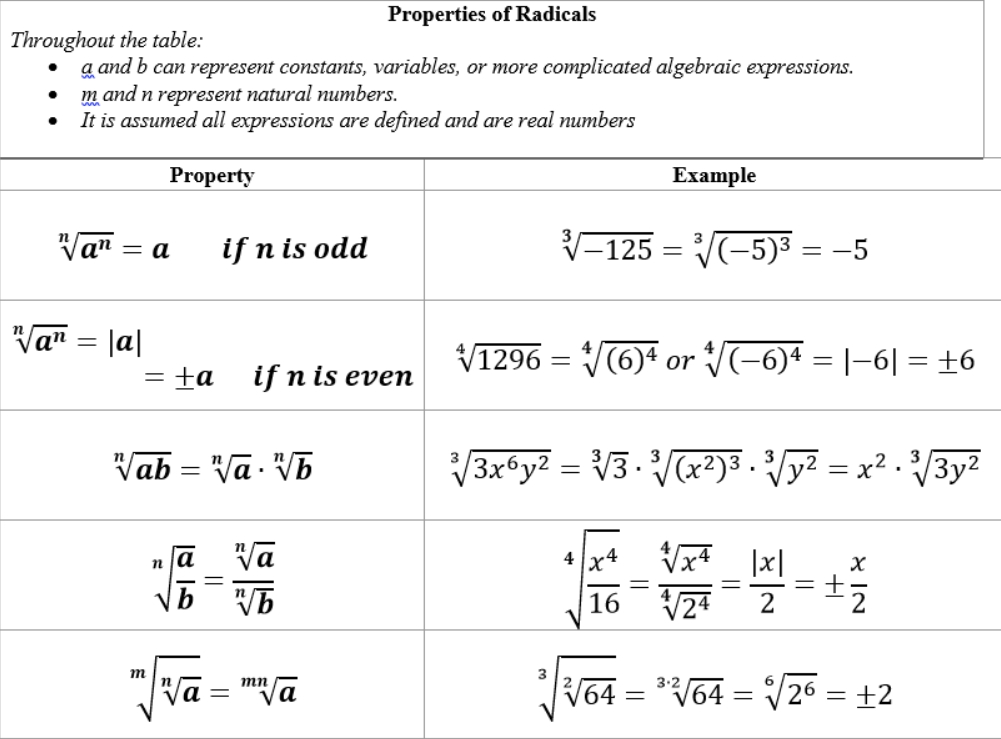
1) Properties of radicals

2) Surds
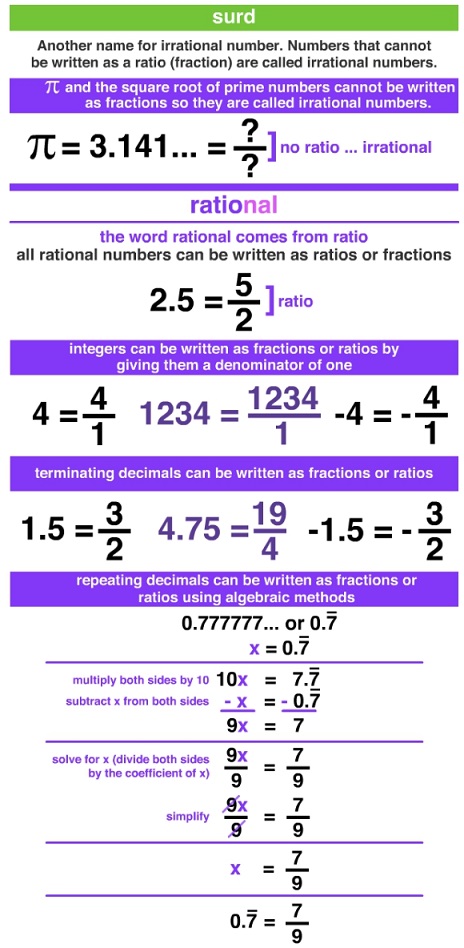
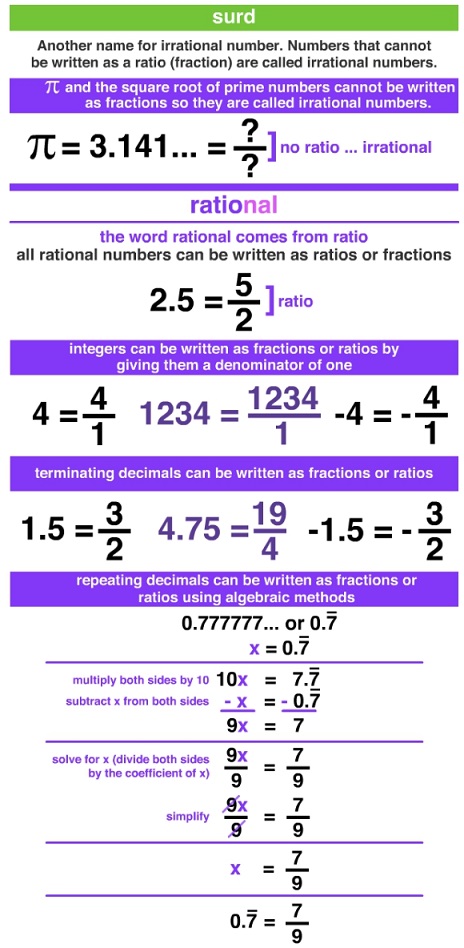
• another name for an irrational number.
• a surd is a real number that can be written as a nonrepeating or nonterminating decimal but not as a fraction because the decimal goes on forever without repeating.
Surds are irrational roots of a rational number. e.g. √6 = a surd ⇒ it can’t be exactly found. Similarly – √7, √8, \(\sqrt[3]{9}\), \(\sqrt[4]{27}\) etc. are all surds.
e.g. \(\frac{(6+\sqrt{2})}{(1-\sqrt{3})}=\frac{(6+\sqrt{2})(1+\sqrt{3})}{(1-\sqrt{3})(1+\sqrt{3})}=\frac{(6+6 \sqrt{3}+\sqrt{2}+\sqrt{6})}{(1-3)}=\frac{(6+6 \sqrt{3} +\sqrt{2}+\sqrt{6})}{-2}\)
GRE surds.jpg [ 137.24 KiB | Viewed 3615 times ]
GRE properties of radicals.jpg [ 184.63 KiB | Viewed 3585 times ]
| Basic operations of indices | |||||
Math operations | Examples | ||||
| \(a^m*a^n=a^{m+n}\) | \(2^3*2^5=2^{3+5}=2^8\) | ||||
| \(\frac{a^m}{a^n}=a^{m-n}\) | \(\frac{2^3}{2^5}=2^{3-5}=2^{-2}\) | ||||
| \((a^m)^n=a^{m*n}\) | \((2^2)^3=2^{2*3}=2^6\) | ||||
| \(x^{-n}=\frac{1}{x^n}\) | \(2^{-2}=\frac{1}{2^2}\) | ||||
| \((\frac{a}{b})^{-n}=(\frac{b}{a})^n\) | \(\frac{1}{2^{-2}}=2\) | ||||
| \(\frac{1}{a^m}=a^{-m}\) | \(\frac{1}{2^3}=2^{-3}\) | ||||
| \(\sqrt[n]{a^m}=(a^m)^{\frac{1}{n}}=a^{\frac{m}{n}}\) | \( \sqrt[3]{2^6}\)\(=(2^6)^{\frac{1}{3}}=2^{\frac{6}{3}}=2^2=4\) | ||||
| \(a^0=1\), if \(a \neq 0\) | \(2^0=1\) | ||||
| \((-a)^{even}=+(a)^{even}\) \(a>0\) | \((-2)^4=-2 \times -2 \times -2 \times -2 = 16\) | ||||
| \((-a)^{odd}=-(a)^{odd}\) \(a>0\) | \(-(2)^3=-(2 \times 2 \times 2)=-8\) | ||||
Note \((a^m)^n \neq a^{m^n}\)
\((2^2)^3 = 2^6=64\)
\(2^{3^2}=2^9=512 \neq 64\)
2) Unit Digit Power
| Unit Digit or Last Digit of a^n | |||||
a\n | 1 | 2 | 3 | 4 | cyclicity |
| 0 | 0 | 0 | 0 | 0 | 1 |
| 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 2 | 4 | 8 | 6 | 4 |
| 3 | 3 | 9 | 7 | 1 | 4 |
| 4 | 4 | 6 | 4 | 6 | 2 |
| 5 | 5 | 5 | 5 | 5 | 1 |
| 6 | 6 | 6 | 6 | 6 | 1 |
| 7 | 7 | 9 | 3 | 1 | 4 |
| 8 | 8 | 4 | 2 | 6 | 4 |
| 9 | 9 | 1 | 9 | 1 | 2 |
Example
Find the unit digit of \(7^{99}\)?
Having a cyclicity of 4 we do have that \(7^{10}\) has a unit digit of 9. This for nine times which is \(7^{90} =\) unit digit of 9 + 9 spots the unit digit is 3
Question to practice about perfect square
https://gre.myprepclub.com/forum/p-23-25-2 ... tml#p43949
https://gre.myprepclub.com/forum/if-x-is-a ... tml#p32890
https://gre.myprepclub.com/forum/qotd-7-n- ... html#p5177
https://gre.myprepclub.com/forum/y-20504.html#p60545
https://gre.myprepclub.com/forum/for-which ... tml#p42721
https://gre.myprepclub.com/forum/units-dig ... tml#p62633
3) Unit Digit of a Square
The square of a number can never end with \(2, 3, 7, 8\) or odd number of zeros.
4) Number rearrangement
When a two-digit number is reversed then the sum of two numbers is always divisible by 11 & the difference of two numbers is always divisible by 9
Let's say we have a number 92
When reversed it will be 29
Sum = 92+29 = \(121\) ----> Divisible by \(11\)
Difference = 92-29 = \(63\) ----> Divisible by \(9\)
5) Square of 5
Suppose that X is a number ending in 5 or 5 itself
\((X5)^2=X(X+1),25\)
\(625^2=62 \times 63\),\(25\)\(=\)\(3906\)\(25\)
You just need to multiply \(62 \times 63\) and add \(25\)
6) Square of any number
\(n^2=(n-d)(n+d)+d^2\)
d is the distance from the nearest multiple of 10.100
\(19^2=(19-1)(19+1)+1^2=18 \times 20+1=361\)
2) Roots
1) Properties of radicals
2) Surds
• another name for an irrational number.
• a surd is a real number that can be written as a nonrepeating or nonterminating decimal but not as a fraction because the decimal goes on forever without repeating.
Surds are irrational roots of a rational number. e.g. √6 = a surd ⇒ it can’t be exactly found. Similarly – √7, √8, \(\sqrt[3]{9}\), \(\sqrt[4]{27}\) etc. are all surds.
- Pure Surd : The surds which are made up of only an irrational number e.g. √6, √7, √8 etc.
- Mixed Surd : Surds which are made up of partly rational and partly irrational numbers e.g. 3√3, \(6^4√27\) etc.
- Rationalization of Surds: In order to rationalize a given surd, multiply and divide by the conjugate of denominator [conjugate of (a + √b) is (a – √b) and vice versa].
e.g. \(\frac{(6+\sqrt{2})}{(1-\sqrt{3})}=\frac{(6+\sqrt{2})(1+\sqrt{3})}{(1-\sqrt{3})(1+\sqrt{3})}=\frac{(6+6 \sqrt{3}+\sqrt{2}+\sqrt{6})}{(1-3)}=\frac{(6+6 \sqrt{3} +\sqrt{2}+\sqrt{6})}{-2}\)
Show: ::
Attachment:
GRE surds.jpg [ 137.24 KiB | Viewed 3615 times ]
Attachment:
GRE properties of radicals.jpg [ 184.63 KiB | Viewed 3585 times ]





